Energetics parameter estimation of jointed rock mass based on Hoek-Brown failure criterion
-
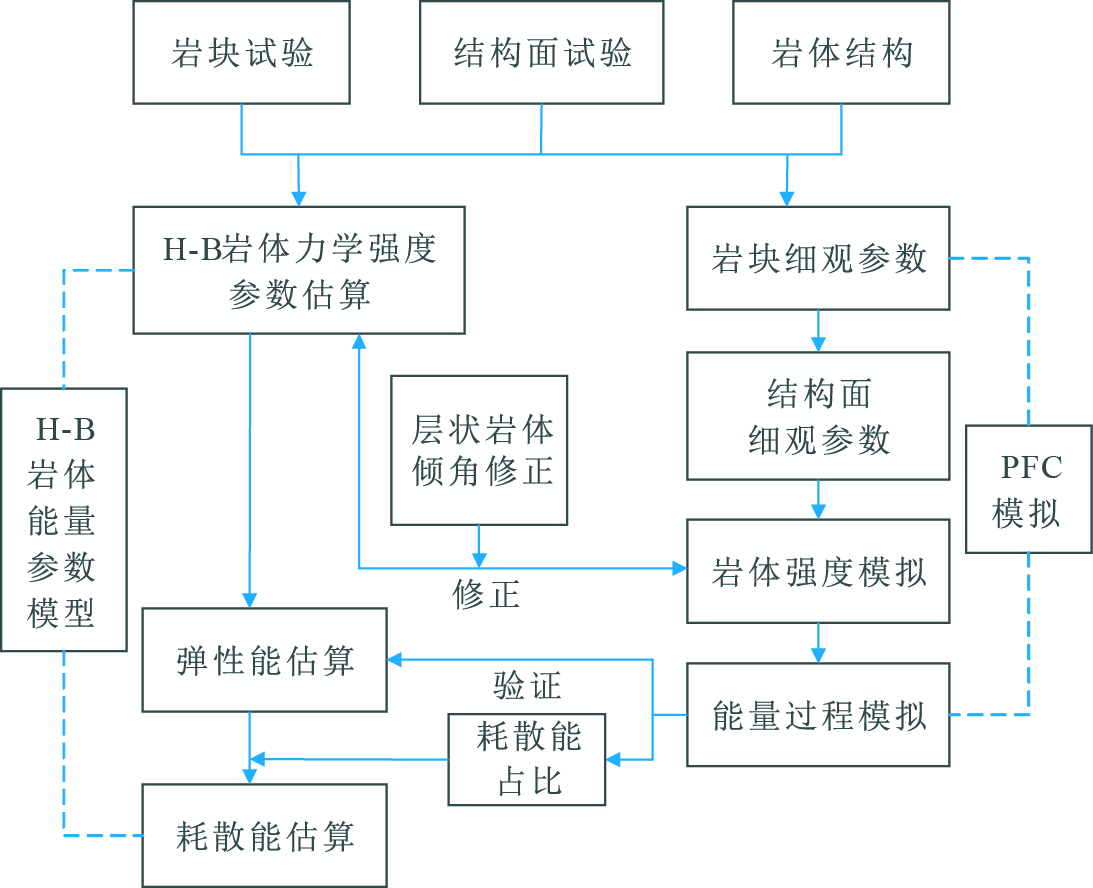
摘要: 岩体变形和破坏可以看作能量耗散和释放的过程。由于节理岩体结构复杂且难以开展室内试验,因此无法通过试验直接求取其能量学参数。基于Hoek-Brown准则和岩石能量理论,提出了节理岩体在临界状态能量学参数的估算方法。针对含贯通节理(或层面)岩体,通过修正岩块单轴抗压强度以体现贯通节理的方向效应。采用PFC3D分别模拟小尺寸岩样(Φ50 mm×100 mm)和大尺寸岩体(Φ2 m×2 m)的三轴压缩试验,通过岩石三轴试验结果拟合岩石数值模拟的细观参数并应用于节理岩体的模拟。根据节理岩体模拟得到应力应变曲线和能量流,验证了Hoek-Brown准则对节理岩体能量参数估算的合理性。Abstract: The deformation and failure of rocks are a process of energy dissipation and release. For the jointed rock masses, the estimation of energetics parameters during loading procedures is a challenge because of their complex structures and difficulties of laboratory tests. The paper proposed a methodology for the energetics parameter estimation of jointed rock masses in critical state based on the Hoek-Brown failure criterion and rock energy theory. For the rock mass with continuous joints (or layers), the orientation effect of continuous joints was reflected by the revised uniaxial compressive strength of rock piece. The PFC3Dnumerical simulation software was used to simulate the triaxial compressive process with small size models of rock pieces (Φ50 mm×100 mm) and big size models of rock masses (Φ2 m×2 m). The mesoscopic parameters of rock numerical model were calibrated by the result of triaxial compression test and applied for the numerical simulation of jointed rock mass. The estimated energetics parameters derived from Hoek-Brown failure criterion were proved to be accurate by the validation with simulated stress strain curves and energy curves.
-
表 1 PFC3D模型细观参数
Table 1. Mesoscopic parameters of PFC30 rock model
细观参数 取值 墙体 法向刚度/(N·m-1) 1.2×109 切向刚度/(N·m-1) 1.2×109 颗粒 法向刚度/(N·m-1) 1.2×108 切向刚度/(N·m-1) 1.2×108 密度/(g·cm-3) 2 650 最小粒径/m 1.0×10-3 粒径比 3.0 摩擦系数 0.8 平行粘结
模型抗拉强度/Pa 2.0×108 内聚力/Pa 5.0×107 有效弹性模量 1.0×109 内摩擦角/(°) 30.0 平滑节理模型
(smooth-joint)法向刚度/(N·m-1) 1.0×1070.1 切向刚度(N/m) 1.0×107 抗拉强度/Pa 2.0×106 内聚力/Pa 5.0×105 摩擦系数 0.3 影响范围 0.1 表 2 含不同倾角贯通节理岩体的三轴抗压强度
Table 2. Triaxial compressive strengths of layered rock mass with different layer dip angles
节理倾角/
(°)PFC模拟
TCS(MPa)σc修正
系数H-B估算
TCS/MPa无 56 1 49.2 15 34 0.61 33.4 30 22 0.39 24.5 45 12 0.21 16.6 60 15 0.27 19.0 75 32 0.57 31.9 90 43 0.77 39.9 表 3 三组优势节理产状和直径分布参数
Table 3. The distribution parameters of orientations and diameters of dominant joint sets
优势组 产状 直径或迹长 分布 倾向/(°) 倾角/(°) k 分布 a 最大/m 最小/m 1 Fisher 120 90 200 power-law 4 2.0 1.2 2 30 20 500 4 2.0 1.2 3 70 45 500 3.2 2.0 1.2 表 4 断续节理岩体峰值强度和弹性模量估算
Table 4. The estimated TCS and elastic modulus of jointed rock masses
节理密度/
(m2/m3)Jv
(条·m-3)GSI mb S a σ1/
MPaE0/GPa PFC3D H-B H-D1 H-D2 0.0 0.0 90 11.89 0.329 0.501 59.8 2.10 97.74 4.47 2.01 0.5 1.59 75 6.96 0.062 0.505 33.2 1.34 39.11 3.47 1.46 2.0 9.87 52 3.06 0.005 0.530 16.7 0.49 10.41 1.73 0.73 4.0 12.57 50 2.85 0.004 0.534 15.8 0.355 9.27 1.54 0.65 6.0 17.98 47 2.56 0.003 0.542 14.4 0.152 7.80 1.27 0.53 表 5 三轴加载试验含断续节理岩体峰值状态的弹性能估算值
Table 5. The estimated elastic energy in the peak stress of jointed rock mass under the triaxial loading
节理密度/
(m2·m-3)弹性能/106J PFC3D 式(9)* 式(2) 0 6.958 5.470 4.760 0.5 2.901 2.288 2.777 2 0.918 1.121 0.754 4 0.864 1.117 0.742 6 0.450 1.139 0.320 *:使用式(9)估算时峰值强度采用H-B准则估算,弹性模量采用H-D准则估算;*:使用式(2)估算时峰值强度和弹性模量均使用PFC3D的模拟结果 -
[1] Hoek E, Brown E T.Practical estimates of rock mass strength[J].International Journal of Rock Mechanics and Mining Sciences, 1997, 34(8):1165-1186. doi: 10.1016/S1365-1609(97)80069-X [2] Cai M, Kaiser P K, Uno H, et al. Estimation of rock mass deformation modulus and strength of jointed hard rock masses using the GSI system[J].International Journal of Rock Mechanics and Mining Sciences, 2004, 41(1):3-19. doi: 10.1016/S1365-1609(03)00025-X [3] Marinos V, Marinos P, Hoek E.The geological strength index:Applications and limitations[J].Bulletin of Engineering Geology and the Environment, 2005, 64(1):55-65. doi: 10.1007/s10064-004-0270-5 [4] Dinc O S, Sonmez H, Tunusluoglu C, et al.A new general empirical approach for the prediction of rock mass strengths of soft to hard rock masses[J].International Journal of Rock Mechanics and Mining Sciences, 2011, 48(4):650-665. doi: 10.1016/j.ijrmms.2011.03.001 [5] Jiang H.A failure criterion for rocks and concrete based on the Hoek-Brown criterion[J].International Journal of Rock Mechanics and Mining Sciences, 2017, 95:62-72. doi: 10.1016/j.ijrmms.2017.04.003 [6] 周广照, 彭云晖, 许思勇, 等.Hoek-Brown准则在岩石强度各向异性评价中的应用[J].地质科技情报, 2017, 36(2):285-292. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dzkjqb201702038 [7] Cai M, Horii H.A constitutive model of highly jointed rock masses[J].Mechanics of Materials, 1992, 13(3):217-246. doi: 10.1016/0167-6636(92)90004-W [8] 宋康明, 姜阳厚, 谭志祥, 等.基于随机森林方法的岩石节理粗糙度系数研究[J].地质科技情报, 2018, 37(3):263-267. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dzkjqb201803035 [9] 金丰年, 蒋美蓉, 高小玲.基于能量耗散定义损伤变量的方法[J].岩石力学与工程学报, 2004, 23(12):1976-1980. doi: 10.3321/j.issn:1000-6915.2004.12.004 [10] 谢和平, 鞠杨, 黎立云, 等.岩体变形破坏过程的能量机制[J].岩石力学与工程学报, 2008, 27(9):1729-1740. doi: 10.3321/j.issn:1000-6915.2008.09.001 [11] Song D, Wang E, Li Z, et al.Energy dissipation of coal and rock during damage and failure process based on EMR[J].International Journal of Mining Science and Technology, 2015, 25(5):787-795. doi: 10.1016/j.ijmst.2015.07.014 [12] 黄达, 黄润秋, 张永兴, 等.粗晶大理岩单轴压缩力学特性的静态加载速率效应及能量机制试验研究[J].岩石力学与工程学报, 2012, 31(2):245-255. doi: 10.3969/j.issn.1000-6915.2012.02.003 [13] 温韬, 刘佑荣, 胡政, 等.高应力区砂岩加卸载条件下能量变化规律及损伤分析[J].地质科技情报, 2015, 34(2):200-206. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dzkjqb201502029 [14] 朱泽奇, 盛谦, 肖培伟, 等.岩石卸围压破坏过程的能量耗散分析[J].岩石力学与工程学报, 2012, 31(2):245-255. doi: 10.3969/j.issn.1000-6915.2012.02.003 [15] 林杭, 李江腾, 曹平.岩石临界应变能释放率测试及其相关规律[J].中南大学学报:自然科学版, 2013, 44(2):714-717. http://d.old.wanfangdata.com.cn/Periodical/zngydxxb201302040 [16] Wasantha P L P, Ranjith P G, Shao S S.Energy monitoring and analysis during deformation of bedded-sandstone:Use of acoustic emission[J].Ultrasonics, 2014, 54(1):217-226. doi: 10.1016/j.ultras.2013.06.015 [17] Potyondy D O, Cundall P A.A bonded-particle model for rock[J].International Journal of Rock Mechanics and Mining Sciences, 2004, 41(8):1329-1364. doi: 10.1016/j.ijrmms.2004.09.011 [18] Potyondy D O.A flat-jointed bonded-particle material for hard rock[C]//Proceedings of the 46th Rock Mechanics/Geomechanics Symposium, Chicago, IL, American Rock Mechanics Association, 2012 [19] 赵忠虎, 谢和平.岩石变形破坏过程中的能量传递和耗散研究[J].四川大学学报:工程科学版, 2008.40(2):26-31. http://d.old.wanfangdata.com.cn/Periodical/scdxxb-gckx200802005 [20] Hoek E, Diederichs M S.Empirical estimation of rock mass modulus[J].International Journal of Rock Mechanics and Mining Sciences, 2006, 43(2):203-215. doi: 10.1016/j.ijrmms.2005.06.005 [21] Bahaaddini M, Sharrock G, Hebblewhite B K.Numerical direct shear tests to model the shear behaviour of rock joints[J].Computers and Geotechnics, 2013, 51(Complete):101-115. https://www.sciencedirect.com/science/article/pii/S0266352X13000293 [22] Chang C, Zoback M D, Khaksar A.Empirical relations between rock strength and physical properties in sedimentary rocks[J].Journal of Petroleum Science and Engineering, 2006, 51(3/4):223-237. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=cdd07ea33a0bb18b7ed228cb59864c8a [23] Rajmeny P K, Singh U K, Rathore S S.A new model to estimate rock mass strength accounting for the scale effect[J].International Journal of Rock Mechanics and Mining Sciences, 2004, 41(6):1013-1021. doi: 10.1016/j.ijrmms.2004.03.008 [24] Schultz R A.Relative scale and the strength and deformability of rock masses[J].Journalof Structural Geology.1996, 18(9):1139-1149. doi: 10.1016/0191-8141(96)00045-4 -





 下载:
下载: