Vertical soil pressure calculation model of rectangular box jacking considering grouting effect
-
摘要: 针对矩形顶管上竖向土压力计算模型研究较少的现状，借助Terzaghi土压力计算理论，结合矩形顶管工程特点建立了考虑注浆作用的矩形顶管竖向土压力计算模型，提出了改进的竖向土压力计算公式；依托苏州某矩形顶管工程中竖向土压力实时监测数据，探究了其变化规律并验证了该计算公式的准确性。研究结果表明：土体中的剪切带从管道外壁两侧产生并沿竖直方向发展，且可贯穿至地表；临界状态下剪切带上的膨胀角完全发挥，以临界内摩擦角及其正弦值计算剪切带上的摩擦系数；不同的注浆压力下，管道上方可能出现“主动土拱”和“被动土拱”。计算值与实测值的对比分析表明，改进后的计算方法能够较好地包络矩形顶管竖向土压力范围。
-
关键词:
- 矩形顶管 /
- 竖向土压力 /
- Terzaghi理论 /
- 注浆作用 /
- 计算模型
Abstract: In view of the current situation that there are few studies on the calculation model of the vertical soil pressure on the rectangular box jacking, the calculation model of the soil pressure on the rectangular box jacking considering the grouting effect is established based on the Terzaghi soil pressure calculation theory and combined with the characteristics of the rectangular box jacking engineering, and an improved calculation formula of the soil pressure on the rectangular box jacking is proposed.Based on the real-time monitoring data of vertical soil pressure in a rectangular box jacking project in Suzhou, the variation rules were explored and the accuracy of the calculation formula was verified.The results show that the shear zone in the soil is generated from both sides of the pipe wall and develops in the vertical direction, and can penetrate to the surface; under the critical state, the frictional angle of the shear zone is fully developed, and the friction coefficient of the shear zone is calculated by the critical internal friction angle and its sinusoidal value; under different grouting pressures, "active soil arch" and "passive soil arch" may appear above the pipeline.The comparative analysis between the calculated value and the measured value shows that the improved calculation method can better envelop the vertical earth pressure range of the rectangular box pipe.-
Key words:
- rectangular box jacking /
- vertical soil pressure /
- Terzaghi theory /
- grouting effect /
- calculation model
-
图 1 Terzaghi土压力计算模型
σh为剪切带处的正应力(kPa)；τf为剪切带处的剪应力(kPa)；q为剪切带上方土柱荷载(kPa); Z为顶管顶部埋深(m); Z1为剪切带上方土柱高度(m); a、b、c为辅助线，分别表示矩形顶管底部、顶部以及剪切带发展高度位置; 其他代号说明同正文
Figure 1. Terzaghi soil pressure calculation model
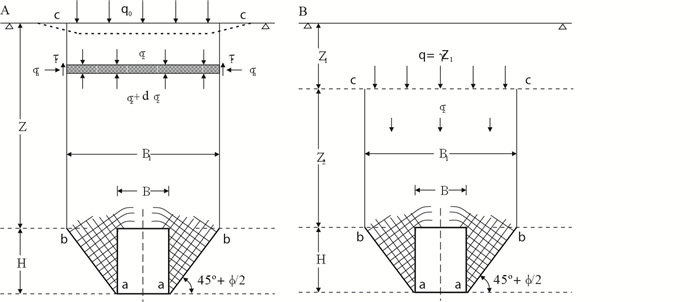
图 2 改进的矩形顶管土压力计算模型(物理量同图 1)
Figure 2. An improved soil pressure calculation model for rectangular box jacking
图 3 顶管隧道所处的地层情况
Figure 3. Strata of tunnel excavated by method of pipe jacking machine
图 4 竖向水土压力监测布点图(单位：m)
Figure 4. Distribution of vertical soil and water pressure monitoring on pipe top
图 5 土压力上下限计算值与实测值对比
Figure 5. Comparison of calculated and measured values of the upper and lower limits of soil pressure
表 1 Terzaghi土拱模型和各个公式中主要对比参数
Table 1. Main comparison parameters in the Terzaghi soil arch model and various formulas
B1 φ K c Terzaghi H(1+2tan α) φ 1 根据实测值确定 GB50332 D1(1+tan α) Ktan φ=0.19 不考虑 不考虑 JMA (D1+0.08)·(sec α+tan α) φ 1 根据实测值确定 PJA D1(sec α+tan α) φ Ka 不考虑 ATV A161 $\sqrt{3 D_{1}}, \varphi=30^{\circ}$ φ/2 K0=0.5 不考虑 ASCE27 D1 Ktan φ=0.11~0.19 根据实测值确定 注：α=45°－ φ/2，B1为计算宽度(m)；D1为管道外径(m)；φ为土体内摩擦角(°)；c为土体黏聚力(kN/m2)；K为土的侧压力系数；K0为静止土压力系数；Ka为主动土压力系数 
下载: 导出CSV
表 2 地层物理力学性质参数
Table 2. Physical and mechanical properties of strata
地层名称 土层厚度/m 重度/(kN·m-3) 压缩模量/MPa 黏聚力/kPa 内摩擦角/(°) 素填土 4.3 19.2 6.12 27.9 16.8 黏土 2.0 19.9 7.37 41.4 15.7 粉质黏土夹粉土 1.3 19.2 6.53 16.8 22.7 粉砂夹粉土 4.4 19.1 9.38 4.6 31.4 粉砂 4.0 19.4 9.70 3.8 33.4
下载: 导出CSV
表 3 注浆加固后地层土体参数计算值
Table 3. Calculation values of soil parameters after grouting reinforcement
地层名称 土层厚度/m 重度/(kN·m-3) 压缩模量/MPa 黏聚力/kPa 内摩擦角/(°) 素填土 4.3 19.2 6.12 27.9 16.8 黏土 2.0 19.9 7.37 41.4 15.7 粉质黏土夹粉土 1.3 19.2 6.53 16.8 22.7 粉砂夹粉土 4.4 19.1 9.38 5.98* 40.82* 粉砂 4.0 19.4 9.70 4.94* 43.42* 注：*代表注浆加固后变化的土体参数值
下载: 导出CSV
-
[1] Ma B, Najafi M. Development and applications of trenchless technology in China[J]. Tunnelling and Underground Space Technology, 2008, 23(4): 476-480. doi: 10.1016/j.tust.2007.08.003 [2] 周浩, 马保松, 赵阳森, 等. 多因素下大断面矩形顶管施工对地层竖向变形影响研究[J]. 隧道建设, 2020, 40(9): 1324-1332. https://www.cnki.com.cn/Article/CJFDTOTAL-JSSD202009013.htm [3] 李剑, 张鹏, 李志宏. 顶管施工润滑泥浆压力引起的土体附加应力计算[J]. 地质科技情报, 2016, 35(2): 28-32. https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201602006.htm [4] 张鹏, 谈力昕, 马保松. 考虑泥浆触变性和管土接触特性的顶管摩阻力公式[J]. 岩土工程学报, 2017, 39(11): 2043-2049. doi: 10.11779/CJGE201711012 [5] 张海丰, 周维, 张鹏, 等. 管土相互作用土箱模型实验设计[J]. 地质科技情报, 2016, 35(4): 219-222. doi: 10.3969/j.issn.1009-6248.2016.04.014 [6] 姜伟, 赵文平, 董威, 等. 大断面矩形顶管周围土压力与顶推力计算方法[J]. 内蒙古科技大学学报, 2019, 38(4): 375-379. https://www.cnki.com.cn/Article/CJFDTOTAL-BTGX201904014.htm [7] 杨仙, 张可能, 黎永索, 等. 深埋顶管顶力理论计算与实测分析[J]. 岩土力学, 2013, 34(3): 757-761. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201303024.htm [8] 李方楠, 沈水龙, 罗春泳. 考虑注浆压力的顶管施工引起土体变形计算方法[J]. 岩土力学, 2012, 33(1): 204-208. doi: 10.3969/j.issn.1000-7598.2012.01.032 [9] 王忠凯, 徐光黎. 盾构施工对既有建(构)筑地基承载力影响及加固土体稳定性分析[J]. 地质科技通报, 2020, 39(4): 109-116. http://dzkjqb.cug.edu.cn/CN/abstract/abstract10006.shtml [10] Terzaghi K. The shearing resistance of saturated soils and the angle between planes of shear[C]//Proc. of Int. Conf. on Smfe. 1936: 1. [11] Zhang H, Zhang P, Zhou W, et al. A new model to predict soil pressure acting on deep burial jacked pipes[J]. Tunnelling and Underground Space Technology, 2016, 60: 183-196. doi: 10.1016/j.tust.2016.09.005 [12] Terazaghi K. Theoretical soil mechanics[M]. New York: John Wiley and Sons, 1945. [13] 中华人民共和国建设部. GB50332-2017给水排水工程管道结构设计规范[S]. 北京: 中国技术出版社, 2017. [14] 上海市政工程设计研究总院. 给水排水工程顶管技术规程[M]. 北京: 中国计划出版社, 2008. [15] 邹超, 姜玉松. 注浆对土体力学性能的影响[C]//中国岩石力学与工程学会锚固与注浆分会. 第四届中国岩石锚固与注浆学术会议论文集. 北京: 中国电力出版社, 2007: 87-91 [16] 何超, 张鹏, 马保松. 拱北隧道管幕工程顶管间距计算分析[J]. 隧道建设, 2019, 39(1): 94-101. https://www.cnki.com.cn/Article/CJFDTOTAL-JSSD201901015.htm -
下载:
点击查看大图
计量
- 文章访问数: 742
- PDF下载量: 900
- 被引次数: 0
