岩溶含水层饱和-非饱和流动与污染物运移数值模拟

郑小康; 杨志兵

doi:10.19509/j.cnki.dzkq.2022.0211

岩溶含水层饱和-非饱和流动与污染物运移数值模拟

doi: 10.19509/j.cnki.dzkq.2022.0211

郑小康^{a, b,},
杨志兵^{a, b, ,}

a.
武汉大学水资源与水电工程科学国家重点实验室, 武汉 430072
b.
武汉大学水工岩石力学教育部重点实验室, 武汉 430072

基金项目:

国家自然科学基金项目 42077177

国家自然科学基金项目 41877203

详细信息

作者简介:
郑小康(1997—)，男，现正攻读水工结构工程专业博士学位，主要从事水文地质研究工作。E-mail：xkzheng@whu.edu.cn

通讯作者:
杨志兵(1982—)，男，教授，博士生导师，主要从事水文地质领域教学和科研工作。E-mail：zbyang@whu.edu.cn

中图分类号: P641.134
计量
- 文章访问数: 680
- PDF下载量: 107
- 被引次数: 0
出版历程
- 收稿日期: 2022-07-05
- 网络出版日期: 2022-11-10

Numerical simulation of saturated-unsaturated groundwater flow and contaminant transport in a karst aquifer

Zheng Xiaokang^{a, b
,},
Yang Zhibing^{a, b
, ,}

a.
State Key Laboratory of Water Resources and Hydropower Engineering Science, Wuhan University, Wuhan 430072, China
b.
Key Laboratory of Rock Mechanics in Hydraulic Structural Engineering of the Ministry of Education, Wuhan University, Wuhan 430072, China

摘要

摘要:
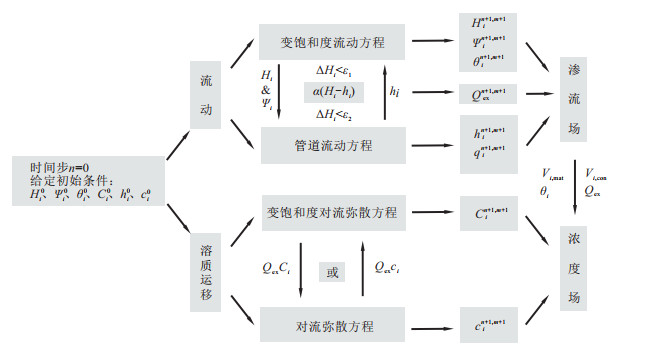
岩溶含水层中的地下水是重要的可饮用水来源, 由于其复杂的水文地质特征, 采用数值模型分析和评价含水层中地下水环境极具挑战性。采用变饱和度流动方程描述含水层基质中的饱和-非饱和流动, 采用管道流方程描述岩溶管道中的层流及紊流流动, 基于流动解采用对流弥散方程分别描述基质与管道中的溶质迁移过程。根据相关实验数据以及MODFLOW-CFP程序的模拟结果对流动模拟的准确性进行验证, 并基于此开展了岩溶管道以及非饱和参数对地下水流动及污染物迁移影响的模拟。结果显示, 数值模型能很好地重现含水层中饱和区与非饱和区以及岩溶管道的地下水动态, 岩溶管道对含水层的渗流场产生了较大的影响。管道中快速流动的特性使得周围的水向其中汇集, 影响了局部流动场, 进而使得泄露的污染物质随水流进入管道, 快速向管道出口处(泉点)迁移, 突破曲线显示管道出口处的污染物浓度响应速度远快于基质中对应的位置, 非饱和参数对管道中污染物浓度的响应速率有着不同程度的影响。建立的模型完善了岩溶含水层中非饱和区地下水流动、污染物运移以及基质-管道流动与溶质运移耦合模拟等方面的工作, 加深了对岩溶含水层地下水动力学以及污染物迁移过程的认识, 对岩溶水文地质及地下水环境等方面具有重要意义。
- 岩溶含水层 /
- 地下水环境 /
- 变饱和度 /
- 地下水流动 /
- 污染物运移
Abstract:
Groundwater in karst aquifers is an important source of drinking water. Due to the complexity of hydrogeological characteristics, it is extremely challenging to analyze and evaluate the groundwater environment in karst aquifers by numerical modeling. In this study, a variable saturation flow equation is used to describe the saturated-unsaturated flow in the aquifer matrix, a pipe flow equation is used to describe the laminar and turbulent flow in karst conduits, and the convective dispersion equation is used to describe solute transport in the matrix and conduits. The accuracy of saturated-unsaturated flow simulation is verified by comparison with experimental data and simulation results from the MODFLOW-CFP code. The numerical model is used to explore the effects of karst conduits and unsaturated parameters on groundwater flow and contaminant transport. These results show that the numerical model can well reproduce the groundwater dynamics in saturated-unsaturated regions and in the karst conduit of the aquifer; furthermore, the karst conduit has a great influence on the seepage field of the aquifer. Relatively rapid flow in the conduit leads to the inflow of surrounding groundwater, affecting the local flow field. As a result, the contaminant is transported through advection in the conduit and is quickly discharged through the outlet(spring). Breakthrough curves show that the response of concentration at the spring is faster than the corresponding location in the matrix. Unsaturated parameters have different effects on the breakthrough characteristics. The model established in this paper improves the simulation methods of groundwater flow, pollutant transport and coupling of matrix-conduit flow with solute transport in the saturated-unsaturated region in karst aquifers. The work deepens the understanding of groundwater dynamics and contaminanttransport in karst aquifers and is thus of great significance to karst hydrogeology and groundwater environment protection.
- karst aquifer /
- groundwater environment /
- variable saturation /
- groundwater flow /
- contaminant transport

HTML全文

图 1 数值模型求解步骤

Figure 1. Strategies for solving numerical models

下载: 全尺寸图片幻灯片

图 2 饱和-非饱和渗流数值模拟自由水面与试验数据^[37]对比

Figure 2. Comparison of the free surface of groundwater in the saturated-unsaturated seepage numerical simulation with the experimental data

下载: 全尺寸图片幻灯片

图 3 地下水水头值分布

Figure 3. Distribution of groundwater hydraulic head

下载: 全尺寸图片幻灯片

图 4 地下水流速分布(a为2 h时刻，b为8 h时刻)

Figure 4. Distribution of groundwater velocity

下载: 全尺寸图片幻灯片

图 5 模型(算例2)

a.平面示意图; b.本研究模型水头值模拟结果；c.MODFLOW-CFP程序水头值模拟结果

Figure 5. A plane diagram of the model (example 2)

下载: 全尺寸图片幻灯片

图 6 岩溶含水层模型剖面示意图

Figure 6. Schematic diagram of the section of the karst aquifer

下载: 全尺寸图片幻灯片

图 7 考虑岩溶管道的基质地下水水头值分布

Figure 7. Distribution of groundwater hydraulic head in the matrix considering karst conduits

下载: 全尺寸图片幻灯片

图 8 考虑岩溶管道的基质地下水流速分布(a为2 h时刻，b为8 h时刻)

Figure 8. Distribution of groundwater velocity in the matrix considering karst conduits

下载: 全尺寸图片幻灯片

图 9 岩溶含水层基质与管道污染物质量浓度突破曲线

Figure 9. Breakthrough curves of the matrix and conduit in the karst aquifer

下载: 全尺寸图片幻灯片

图 10 含水层参数对管道出口污染物质量浓度影响

Figure 10. Influence of hydrogeological parameters on solute concentration at the outlet of conduit

下载: 全尺寸图片幻灯片

表 1 算例1含水层参数取值

Table 1. Parameters of the modeled aquifer (example 1)

序号	参数名称	取值
1	饱和渗透系数K/(m·d^-1)	8.40
2	孔隙度n	0.3
3	最大含水率θ^s	0.3
4	残余含水率θ^r	0.01
5	van Genuchten参数a_v/m^-1	3.3
6	van Genuchten参数n_v	4.1
7	贮水率S_s/m^-1	0.0

下载: 导出CSV

表 2 不同情景下待评价参数取值范围

Table 2. Range of under-evaluation parameters in different scenarios

情景	参数名称	取值范围	系列数
1	孔隙度n/饱和含水率θ^s	0.15~0.40	5
2	残余含水率θ^r	0.01~0.10	5
3	van Genuchten参数a_v/m^-1	0.1~8	5
4	van Genuchten参数n_v	1.5~5	5
5	基质饱和渗透系数K/(m·d^-1)	1.0~20	5
6	管道直径d/m	0.02~0.07	5
7	管道弯曲度τ	1~2	5
8	管壁水力传导度α/(m·d^-1)	0.1~5	5

下载: 导出CSV

参考文献(37)

[1]	Ford D C, Williams P W. Karst hydrogeology and geomorphology[M]. Chichester: John Wiley & Sons Ltd, 2007.
[2]	Goldscheider N, Chen Z, Auler A S, et al. Global distribution of carbonate rocks and karst water resources[J]. Hydrogeology Journal, 2020, 28(5): 1661-1677. doi: 10.1007/s10040-020-02139-5
[3]	Olarinoye T, Gleeson T, Marx V, et al. Global karst springs hydrograph dataset for research and management of the world's fastest-flowing groundwater[J]. Scientific Data, 2020, 7(1): 1-9. doi: 10.1038/s41597-019-0340-y
[4]	王喆. 岩溶地下水系统演化的数值模拟[J]. 地质科技情报, 2013, 32(4): 201-206. https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201304032.htm Wang Z. Numerical simulation of the karst groundwater system evolution[J]. Geological Science and Technology Information, 2013, 32(4): 201-206 (in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201304032.htm
[5]	Zeng S B, Jiang Y J, Liu Z H. Assessment of climate impacts on the karst-related carbon sink in SW China using MPD and GIS[J]. Global and Planetary Change, 2016, 144: 171-181. doi: 10.1016/j.gloplacha.2016.07.015
[6]	Shen T M, Li W, Pan W Z, et al. Role of bacterial carbonic anhydrase during CO₂ capture in the CO₂-H₂O-carbonate system[J]. Biochemical Engineering Journal, 2017, 123: 66-74. doi: 10.1016/j.bej.2017.04.003
[7]	张春来, 黄芬, 蒲俊兵, 等. 中国岩溶碳汇通量估算与人工干预增汇途径[J]. 中国地质调查, 2021, 8(4): 40-52. doi: 10.19388/j.zgdzdc.2021.04.05 Zhang C L, Huang F, Pu J B, et al. Estimation of karst carbon sink fluxes and manual intervention to increase carbon sinks in China[J]. Geological Survey of China, 2021, 8(4): 40-52 (in Chinese with English abstract). doi: 10.19388/j.zgdzdc.2021.04.05
[8]	罗维均, 杨开萍, 王彦伟, 等. 喀斯特地区不同岩土组构对岩溶碳通量的影响[J]. 地质科技通报, 2022, 41(3): 208-214. doi: 10.19509/j.cnki.dzkq.2022.0088 Luo W J, Yang K P, Wang W Y, et al. Influence of different rock-soil fabrics on carbonate weathering carbon sink flux in karst regions[J]. Bulletin of Geological Science and Technology, 2022, 41(3): 208-214 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0088
[9]	Song K, Yang G X, Wang F, et al. Application of geophysical and hydrogeochemical methods to the protection of drinking groundwater in karst regions[J]. International Journal of Environmental Research and Public Health, 2020, 17(10): 3627. doi: 10.3390/ijerph17103627
[10]	陈静, 罗明明, 廖春来, 等. 中国岩溶湿地生态水文过程研究进展[J]. 地质科技情报, 2019, 38(6): 221-230. doi: 10.19509/j.cnki.dzkq.2019.0626 Chen J, Luo M M, Liao C L, et al. Review of Eco-hydrological Process in Karst Wetlands of China[J]. Geological Science and Technology Information, 2019, 38(6): 221-230 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2019.0626
[11]	曾斌, 陈植华, 邵长杰, 等. 基于地下水流系统理论的岩溶隧道涌突水来源及路径分析[J]. 地质科技通报, 2022, 41(1): 99-108. doi: 10.19509/j.cnki.dzkq.2022.0006 Zeng B, Chen Z H, Shao C J, et al. Analysis of source and path of water inrush in karst tunnel based on the theory of groundwater flow system[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 99-108 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0006
[12]	Zhu Z, Wang J X, Hu M G, et al. Geographical detection of groundwater pollution vulnerability and hazard in karst areas of Guangxi Province, China[J]. Environmental Pollution, 2019, 245: 627-633. doi: 10.1016/j.envpol.2018.10.017
[13]	Jeannin P Y, Artigue G, Butscher C, et al. Karst modelling challenge 1: Results of hydrological modelling[J]. Journal of Hydrology, 2021, 600: 126508. doi: 10.1016/j.jhydrol.2021.126508
[14]	Chang Y, Wu J C, Jiang G H, et al. Identification of the dominant hydrological process and appropriate model structure of a karst catchment through stepwise simplification of a complex conceptual model[J]. Journal of Hydrology, 2017, 548: 75-87. doi: 10.1016/j.jhydrol.2017.02.050
[15]	Zhou B Q, Yang Z B, Hu R, et al. Assessing the impact of tunnelling on karst groundwater balance by using lumped parameter models[J]. Journal of Hydrology, 2021, 599: 126375.
[16]	Doummar J, Sauter M, Geyer T. Simulation of flow processes in a large scale karst system with an integrated catchment model (Mike She): Identification of relevant parameters influencing spring discharge[J]. Journal of Hydrology, 2012, 426: 112-123.
[17]	Mayaud C, Wagner T, Benischke R, et al. Understanding changes in the hydrological behaviour within a karst aquifer (Lurbach system, Austria)[J]. Carbonates and Evaporites, 2016, 31(4): 357-365.
[18]	Clement T P, Wise W R, Molz F J. A physically based, two-dimensional, finite-difference algorithm for modeling variably saturated flow[J]. Journal of Hydrology, 1994, 161(1/4): 71-90.
[19]	Liedl R, Sauter M, Hückinghaus D, et al. Simulation of the development of karst aquifers using a coupled continuum pipe flow model[J]. Water Resources Research, 2003, 39(3): 1057.
[20]	Shoemaker W B, Kuniansky E L, Birk S, et al. Documentation of a conduit flow process (CFP) for MODFLOW-2005[M]. Reston, Va: US Department of the Interior, US Geological Survey, 2008.
[21]	Reimann T, Giese M, Geyer T, et al. Representation of water abstraction from a karst conduit with numerical discrete-continuum models[J]. Hydrology and Earth System Sciences, 2014, 18(1): 227-241.
[22]	焦友军, 潘晓东, 曾洁, 等. 岩溶管道结构影响泉流量变化的数值模拟研究[J]. 中国岩溶, 2017, 36(5): 736-742. https://www.cnki.com.cn/Article/CJFDTOTAL-ZGYR201705018.htm Jiao Y J, Pan X D, Zeng J, et al. Numerical modeling of the influence of karst-conduit structure on variation of spring flow[J]. Carsologica Sinica, 2017, 36(5): 736-742 (in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-ZGYR201705018.htm
[23]	Giese M, Reimann T, Bailly Comte V, et al. Turbulent and laminar flow in karst conduits under unsteady flow conditions: Interpretation of pumping tests by discrete conduit-continuum modeling[J]. Water Resources Research, 2018, 54(3): 1918-1933.
[24]	Shu L C, Zou Z K, Li F L, et al. Laboratory and numerical simulations of spatio-temporal variability of water exchange between the fissures and conduits in a karstic aquifer[J]. Journal of Hydrology, 2020, 590: 125219.
[25]	Zheng X K, Yang Z B, Wang S, et al. Evaluation of hydrogeological impact of tunnel engineering in a karst aquifer by coupled discrete-continuum numerical simulations[J]. Journal of Hydrology, 2021, 597: 125765.
[26]	de Rooij R, Perrochet P, Graham W. From rainfall to spring discharge: Coupling conduit flow, subsurface matrix flow and surface flow in karst systems using a discrete continuum model[J]. Advances in Water Resources, 2013, 61: 29-41.
[27]	Malenica L, Gotovac H, Kamber G, et al. Groundwater flow modeling in karst aquifers: Coupling 3d matrix and 1d conduit flow via control volume isogeometric analysis-experimental verification with a 3d physical model[J]. Water, 2018, 10(12): 1787.
[28]	Zheng C M, Wang P P. MT3DMS: A modular three-dimensional multispecies transport model for simulation of advection, dispersion, and Chemical Reactions of Contaminants in Groundwater Systems: Documentation and user's guide, DTIC Document[M]. Tuscaloosa, Alamaba: University of Alabama, 1999.
[29]	Spiessl S M, Prommer H, Licha T, et al. A process-based reactive hybrid transport model for coupled discrete conduit continuum systems[J]. Journal of Hydrology, 2007, 347(1/2): 23-34.
[30]	Chen Y F, Yu H, Ma H Z, et al. Inverse modeling of saturated-unsaturated flow in site-scale fractured rocks using the continuum approach: A case study at Baihetan dam site, Southwest China[J]. Journal of Hydrology, 2020, 584: 124693.
[31]	Chen Y F, Ye Y K, Hu R, et al. Modeling unsaturated flow in fractured rocks with scaling relationships between hydraulic parameters[J/OL]. Journal of Rock Mechanics and Geotechnical Engineering, 2022. https//doi.org/10.1016/j.jrmge.2022.02.008.
[32]	Richards L A. Capillary conduction of liquids through porous mediums[J]. Physics, 1931, 1(5): 318-333.
[33]	Vennard J K, Street R L. Elementary fluid mechanics[M]. New York: John Wiley, 1975.
[34]	Van Genuchten M T. A closed form equation for predicting the hydraulic conductivity of unsaturated soils[J]. Soil Science Society of America Journal, 1980, 44(5): 892-898.
[35]	Horlacher H B, Lüdecke H J. Strömungsberechnung für Rohrsysteme[M]. Ehningen, Germany: Springer-Verlag, 1992.
[36]	Celia M A, Bouloutas E T, Zarba R L. A general mass conservative numerical solution for the unsaturated flow equation[J]. Water Resources Research, 1990, 26(7): 1483-1496.
[37]	Vauclin M, Khanji D, Vachaud G. Experimental and numerical study of a transient, two dimensional unsaturated saturated water table recharge problem[J]. Water Resources Research, 1979, 15(5): 1089-1101.