基于深度学习的大地电磁二维反演方法

摘要:
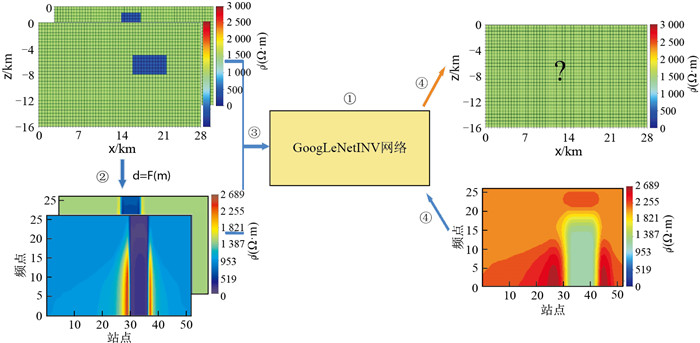
如何通过大地电磁测深反演方法来提高数据解释的精度一直都是大地电磁测深研究领域的重要课题。针对大地电磁传统反演方法存在的初始模型依赖、易陷入局部最优的问题，提出了一种基于深度学习的大地电磁二维反演方法。该方法首先设计GoogLeNetINV神经网络；接着构造多种地电模型，在TM模式下通过正演得到视电阻率数据，组成训练数据集；然后用训练数据集训练该神经网络并调整网络参数；最后，将视电阻率数据输入已训练好的GoogLeNetINV神经网络直接得到反演结果。实验结果表明，该方法能快速、准确地反演出“未学习”过地电模型的位置和电阻率数据，具有较好的泛化能力；使用噪声数据测试仍能取得良好的反演结果，有一定的抗噪声能力。将该神经网络应用于Bendigo Zone实际数据资料处理中，反演得到的电阻率模型与地震解释一致，因此基于深度学习的大地电磁反演方法能有效解决大地电磁反演问题。
- 深度学习 /
- 大地电磁反演 /
- 神经网络 /
- GoogLeNetINV
Abstract: Objective
The inversion of magnetotelluric sounding data to improve the accuracy of data interpretation has always been an essential topic in magnetotelluric sounding.
Methods
To address the problems of traditional magnetotelluric inversion methods, such as the dependence of the initial model and the ease of falling into a local optimum, this paper proposes a magnetotelluric inversion method based on deep learning.The method begins with the design of the GoogLeNetINV neural network. Then, various geoelectric models are constructed, and apparent resistivity data are extracted via forward modelling in the TM mode, constituting the training dataset. Additionally, the neural network is trained with the training dataset, and the network parameters are adjusted. Finally, the apparent resistivity data are input into the trained GoogLeNetINV neural network to directly obtain the inversion result.
Results
The experimental results reveal that the location and resistivity data of the "unlearned" geoelectric model can be inverted quickly and accurately, and the model has good generalization ability. The use of noise data can still yield good inversion results and a certain anti-noise ability.
Conclusion
The neural network is applied to the field data processing of the Bendigo Zone, and the resistivity model derived through inversion is consistent with the seismic interpretation. Consequently, the magnetotelluric inversion method based on deep learning can effectively solve the magnetotelluric inversion problem.
- deep learning /
- magnetotelluric inversion /
- neural network /
- GoogLeNetINV
注释:

所有作者声明不存在利益冲突。

HTML全文

图 1 基于深度学习的大地电磁反演过程(①~④为反演步骤)

Figure 1. Magnetotelluric inversion process based on deep learning

下载: 全尺寸图片幻灯片

图 2 Inception结构

Figure 2. Inception structure

下载: 全尺寸图片幻灯片

图 3 GoogLeNetINV神经网络结构

Figure 3. GoogLeNetINV neural network structure

下载: 全尺寸图片幻灯片

图 4 正演观测系统和模型几何设计

a.观测系统设计；b.模型几何设计

Figure 4. Geometric design of the forward observation system and model

下载: 全尺寸图片幻灯片

图 5 部分验证集样本反演结果

a. 6×6(3 000 Ω·m)；b. 5×10(200 Ω·m)；c. 3层4×8(2 500 Ω·m)；d. 8×4(200 Ω·m)；e. 5×10(2 500 Ω·m)；f. 6×6(500 Ω·m)

Figure 5. Inversion results for the partial validation set samples

下载: 全尺寸图片幻灯片

图 6 实验1反演结果

a. 10×5组合模型(500 Ω·m)；b. 10×5与3层4×8组合模型(3 000 Ω·m); c. 3层4×8组合模型(3 000 Ω·m)

Figure 6. Inversion results of experiment one

下载: 全尺寸图片幻灯片

图 7 实验2反演结果

a. 7×7(2 300 Ω·m); b. 4层4×8(700 Ω·m)

Figure 7. Inversion results of experiment two

下载: 全尺寸图片幻灯片

图 8 加入0%，3%和5%高斯白噪声的视电阻率

a.未加噪声的视电阻率; b.加入3%高斯白噪声的视电阻率; c.加入5%高斯白噪声的视电阻率

Figure 8. Apparent resistivity with 0%, 3% and 5% white Gaussian noise

下载: 全尺寸图片幻灯片

图 9 加入高斯白噪声的反演结果

a. 4层4×8(200 Ω·m)3%高斯白噪声；b. 6×12(2 500 Ω·m)3%高斯白噪声; c. 7×7(3 000 Ω·m)3%高斯白噪声; d. 4层4×8(200 Ω·m)5%高斯白噪声; e. 6×12(2 500 Ω·m)5%高斯白噪声；f. 7×7(3 000 Ω·m)5%高斯白噪声

Figure 9. Inversion results with white Gaussian noise

下载: 全尺寸图片幻灯片

图 10 卷积神经网络(CNN)反演结果^[14]

a.低阻异常浅部反演; b. 低阻异常中部反演; c.低阻异常深部反演; d. 地堑浅部反演; e. 地堑中部反演; f.地堑深部反演

Figure 10. Inversion results of the convolutional neural network (CNN)

下载: 全尺寸图片幻灯片

图 11 GoogLeNetINV神经网络反演结果

a.低阻异常浅部反演; b.低阻异常中部反演; c.低阻异常深部反演; d. 地堑浅部反演; e. 地堑中部反演; f.地堑深部反演

Figure 11. Inversion results of the GoogLeNetINV neural network

下载: 全尺寸图片幻灯片

图 12 区域地质图(据文献[22]修改)

RR为远程参考站点；MT测深用黄色圆圈表示，圆圈上数字代表测点编号；Cayley等^[23]于2011年绘制的MT地震剖面线06GAV-02和06GAV-03用蓝色粗线表示。地质走向显示在图左下方，主要走向近南北向，约N5°W，次要方向为北东向，约为N40°E。AVF、CTF、MFF、WLF、HFZ、MWF均为断层代号，图 14同

Figure 12. Regional geological map

下载: 全尺寸图片幻灯片

图 13 实际数据反演结果对比

a. GoogLeNetINV神经网络反演; b. NLCG代码反演结果^[22]，▽为取样点

Figure 13. Comparison of inversion results for field data

下载: 全尺寸图片幻灯片

图 14 地震偏移剖面及其解释^[24]

a. 地震偏移剖面(06GAV-02); b. 地震解释

Figure 14. Seismic migrated section and its interpretation

下载: 全尺寸图片幻灯片

表 1 GoogLeNetINV神经网络反演参数设置

Table 1. GoogLeNetINV neural network inversion parameter settings

分类	参数设置	GoogLeNetINV神经网络
数据集	训练集	7 190
数据集	验证集	1 797
网络设置	学习率	η=0.001
	激活函数	ReLu
	优化器	Adam
	L2正则化	λ=0.003
	Dropout	0.5
训练过程	epochs	3 000
训练过程	batch size	300

下载: 导出CSV

表 2 GoogLeNetINV神经网络反演及预测所需时间

Table 2. Training and prediction times of the GoogLeNetINV neural network

训练时间/s	3 000
预测时间/s	1

下载: 导出CSV

参考文献(25)

[1]	陈小斌, 赵国泽, 汤吉, 等. 大地电磁自适应正则化反演算法[J]. 地球物理学报, 2005, 48(4): 937-946. https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX202010025.htm CHEN X B, ZHAO G Z, TANG J, et al. An adaptive regularized inversion algorithm for magnetotelluric data[J]. Chinese Journal of Geophysics, 2005, 48(4): 937-946. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-DQWX202010025.htm
[2]	罗曦, 柳建新, 童孝忠. 基于阻尼高斯-牛顿法的MT二维正则化反演[C]//佚名. 中国地球物理学会第二十七届年会论文集. [出版地不详]: [出版社不详], 2011: 315. LUO X, LIU J X, TONG X Z. 2D MT regularization inversion based on damped Gauss-Newton optimization algorithm[C]//Anon. Proceedings of the 27th Annual Meeting of Chinese Geophysical Society. [S. l. ]: [s. n. ], 2011: 315. (in Chinese)
[3]	SU Y, YIN C, LIU Y, et al. 2D magnetotelluric sparse regularization inversion based on curvelet transform[J]. Chinese Journal of Geophysics, 2021, 64(1): 314-326.
[4]	CONSTABLE S C, PARKER R L, CONSTABLE C G. Occam's inversion: A practical algorithm for generating smooth models from electromagnetic sounding data[J]. Geophysics, 1987, 52(3): 289-300. doi: 10.1190/1.1442303
[5]	WEI H, ZHOU H W. Least-squares seismic inversion with stochastic conjugate gradient method[J]. Journal of Earth Science, 2015, 26(4): 463-470. doi: 10.1007/s12583-015-0553-8
[6]	YANG H, WANG J L, WU J S, et al. Constrained joint inversion of magnetotelluric and seismic data using simulated annealing algorithm[J]. Chinese Journal of Geophysics, 2002, 45(5): 764-776. doi: 10.1002/cjg2.290
[7]	何一鸣, 薛国强, 赵炀. 基于量子行为粒子群算法的航空瞬变电磁拟二维反演技术[J]. 地球科学与环境学报, 2020, 42(6): 722-730. https://www.cnki.com.cn/Article/CJFDTOTAL-XAGX202006003.htm HE Y M, XUE G Q, ZHAO Y. Quasi-2D stochastic inversion of airbone transient eletromagnetic data based on quantum-behaved particle swarm optimization algorithm[J]. Journal of Earth Sciences and Environment, 2020, 42(6): 722-730. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-XAGX202006003.htm
[8]	熊杰, 孟小红, 刘彩云, 等. 基于差分进化的大地电磁反演[J]. 物探与化探, 2012, 36(3): 448-451. https://www.cnki.com.cn/Article/CJFDTOTAL-WTYH201203026.htm XIONG J, MENG X H, LIU C Y, et al. Magnetotelluric inversion based on differential evolution[J]. Geophysical and Geochemical Exploration, 2012, 36(3): 448-451. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-WTYH201203026.htm
[9]	杨灿, 刘磊磊, 张遗立, 等. 基于贝叶斯优化机器学习超参数的滑坡易发性评价[J]. 地质科技通报, 2022, 41(2): 228-238. doi: 10.19509/j.cnki.dzkq.2022.0059 YANG C, LIU L L, ZHANG Y L, et al. Machine learning based on landslide susceptibility assessment with Bayesian optimized the hyperparameters[J]. Bulletin of Geological Science and Technology, 2022, 41(2): 228-238. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.2022.0059
[10]	MONTAHAEI M, OSKOOI B. Magnetotelluric inversion for azimuthally anisotropic resistivities employing artificial neural networks[J]. Acta Geophysica, 2014, 62(1): 12-43. doi: 10.2478/s11600-013-0164-7
[11]	WANG H, LIU M, XI Z, et al. Magnetotelluric inversion based on BP neural network optimized by genetic algorithm[J]. Chinese Journal of Geophysics, 2018, 61(4): 1563-1575.
[12]	LIU Z, CHEN H, REN Z, et al. Deep learning audio magnetotellurics inversion using residual-based deep convolution neural network[J]. Journal of Applied Geophysics, 2021, 188: 104309. doi: 10.1016/j.jappgeo.2021.104309
[13]	LIU W, XI Z, WANG H, et al. Two-dimensional deep learning inversion of magnetotelluric sounding data[J]. Journal of Geophysics and Engineering, 2021, 18(5): 627-641. doi: 10.1093/jge/gxab040
[14]	廖晓龙, 张志厚, 姚禹, 等. 基于卷积神经网络的大地电磁反演[J]. 中南大学学报(自然科学版), 2020, 51(9): 2546-2557. https://www.cnki.com.cn/Article/CJFDTOTAL-ZNGD202009020.htm LIAO X L, ZHANG Z H, YAO Y, et al. Magnetotelluric inversion based on convolutional neural network[J]. Journal of Central South University(Science and Technology), 2020, 51(9): 2546-2557. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-ZNGD202009020.htm
[15]	YANG N, ZHANG Z, YANG J, et al. A convolutional neural network of GoogLeNet applied in mineral prospectivity prediction based on multi-source geoinformation[J]. Natural Resources Research, 2021, 30(6): 3905-3923. doi: 10.1007/s11053-021-09934-1
[16]	薛瑞洁, 熊杰, 张月, 等. 基于卷积神经网络的磁异常反演[J]. 现代地质, 2023, 37(1): 173-183. https://www.cnki.com.cn/Article/CJFDTOTAL-XDDZ202301019.htm XUE R J, XIONG J, ZHANG Y, et al. Inversion of magnetic anomaly based on convolutional neural network[J]. Geoscience, 2023, 37(1): 173-183. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-XDDZ202301019.htm
[17]	JIANG F B, DAI Q W, DONG L. Ultra-high density resistivity nonlinear inversion based on principal component-regularized ELM[J]. Chinese Journal of Geophysics, 2015, 58(9): 3356-3369.
[18]	陈麒玉, 刘刚, 何珍文, 等. 面向地质大数据的结构-属性一体化三维地质建模技术现状与展望[J]. 地质科技通报, 2020, 39(4): 51-58. doi: 10.19509/j.cnki.dzkq.2020.0407 CHEN Q Y, LIU G, HE Z W, et al. Current situation and prospect of structure-attribute integrated 3D geological modeling technology for geological big data[J]. Bulletin of Geological Science and Technology, 2020, 39(4): 51-58. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.2020.0407
[19]	王权, 邹艳红. 基于轮廓线层间形态插值的三维地质隐式曲面重构[J]. 地质科技通报, 2023, 42(5): 293-300. doi: 10.19509/j.cnki.dzkq.tb20220003 WANG Q, ZOU Y H. Three-dimensional geological implicit surface reconstruction based on intermediate contour morphological interpolation[J]. Bulletin of Geological Science and Technology, 2023, 42(5): 293-300. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.tb20220003
[20]	LEE S K, KIM H J, SONG Y, et al. MT2DInvMatlab: A program in MATLAB and FORTRAN for two-dimensional magnetotelluric inversion[J]. Computers & Geosciences, 2009, 35(8): 1722-1734.
[21]	陈冠宇, 安凯, 李向. 基于卷积神经网络的不良地质体识别与分类[J]. 地质科技情报, 2016, 35(1): 205-211. https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201601032.htm CHEN G Y, AN K, LI X. Identification and classification of adverse geological body based on convolution neural networks[J]. Geological Science and Technology Information, 2016, 35(1): 205-211. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201601032.htm
[22]	LEE S K, LEE T J, TOSHIHIRO U, et al. Magnetotelluric measurements along a reflection seismic profile: Reprocessing and reinterpretation of MT data for crustal-scale electric resistivity structure in central Victoria, Australia[J]. Geosciences Journal, 2013, 17(2): 289-299.
[23]	CAYLEY R A, KORSCH R J, MOORE D H. Crustal architecture of central Victoria: Results from the 2006 deep crustal reflection seismic survey[J]. Australian Journal of Earth Sciences, 2011, 58(2): 113-156. doi: 10.1080/08120099.2011.543151
[24]	RODI W L, MACKIE R L. Nonlinear conjugate gradients algorithm for 2-D magnetotelluric inversion[J]. Geophysics, 2001, 66(1): 174-187. doi: 10.1190/1.1444893
[25]	VANDENBERG A H M, WILLMAN C E. The Tasman fold belt system in Victoria[J]. Geological Survey of Victoria, 2003, 48(3): 267-297.