Mixing effect and skin effect on radical solute transport around an injection well
-
摘要:
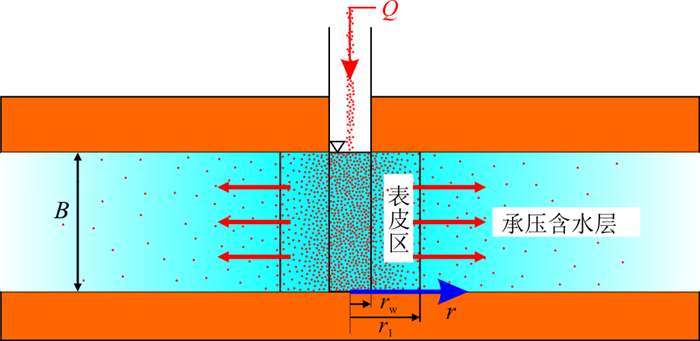
注水井理论模型研究一直是水文地质学领域的热点问题。充分考虑含水层的非均质性，利用两区MIM(Mobile-Immobile)对流扩散模型来描述溶质在含水层中的运移过程，同时考虑表皮效应和井筒混合效应，建立一种描述注入井附近含水层溶质径向运移的动力学模型。并采用Laplace变换、Stehfest数值逆变换得到了该动力学模型的半解析解。然后改变表皮区的有效孔隙度和径向弥散度以及井筒的半径，来分析固定观测点的溶质穿透曲线和溶质浓度分布曲线的变化规律。研究表明，井筒混合效应和表皮效应对穿透曲线、溶质径向运移过程和影响区域均有着非常显著的影响。在考虑井筒混合效应时，井筒半径越大，井筒效应越明显。而表皮区域有效孔隙度越大，溶质的迁移扩展速率越小；径向弥散度越大，观测点的溶质浓度曲线越陡峭，表明该点的溶质浓度变化速率较快，且能更早达到稳定值。与前人研究相比，本研究模型能更好地描述注水井中的溶质径向弥散过程。
Abstract:Objective The conceptual model of the single-well push test is a hot topic in groundwater hydrogeology.
Methods In this study, a new mathematical model was developed for radial solute transport in an aquifer near injection wells. The heterogeneity of the aquifer was considered, and the MIM (Mobile-Immobile) convective diffusion model was used to describe the solute transport process in the aquifer. The skin effect and mixing effect are also included in this conceptual model. The semi-analytical solution was derived by using the Laplace transform and Stehfest numerical inverse transform methods. The influence of effective porosity and radial dispersion of the skin zone and the radius of the wellbore on the solute breakthrough curves (BTCs) of a fixed observation point and solute concentration distribution curves at given times were investigated.
Results Results show that wellbore mixing and skin effects have significant impacts on BTCs, solute radial transport processes and the influence area. The larger the radius of the wellbore is, the more obvious the wellbore mixing effect is. For the skin zone, a larger porosity leads to a smaller velocity of solute migration. The larger the radial dispersion is, the steeper the solute concentration curve of the observation point is, indicating that the solute concentration changes at a faster rate and can reach a stable value earlier.
Conclusion Compared with previous studies, this model can better describe the solute radial dispersion process near the injection wells.
-
Key words:
- skin effect /
- mixing effect /
- injection well /
- radial solute transport
-
图 1 注水井溶质径向迁移概念模型
B为承压层厚度；Q为注入量；γw为注入井半径；r1为表皮区+注入井半径；r为离井筒中心的径向距离
Figure 1. Conceptual model of radial solute transport in a single push well
图 2 不同观测点A、B的穿透曲线半解析解和数值解对比结果
Figure 2. Comparison of the semianalytical solution and numerical solution of BTCs at observation points A and B
图 3 不同表皮区有效孔隙度(θ1m)时观测点A的穿透曲线(a)及溶质浓度径向分布曲线(b)
Figure 3. Breakthrough curves of observation point A (a) and concentration distribution curves (b) for different effective porosities (θ1m) in the skin zone
图 4 不同表皮区径向弥散度(α1)时观测点A的穿透曲线(a)及溶质浓度径向分布曲线(b)
Figure 4. Breakthrough curves of observation point A (a), and concentration distribution curves (b) for different radical dispersivities (α1) in the skin zone
图 5 不同注入井半径(rw)时观测点A的穿透曲线
Figure 5. Breakthrough curves of observation point A for different wellbore radii (rw)
图 6 当t=5 h时(a)和t=30 h时(b)不同注入井半径(rw)的溶质浓度径向分布曲线
Figure 6. Concentration distribution curves for different well radii (rw) of wellbore when t=5 h (a) and t=30 h (b)
表 1 无量纲参数定义
Table 1. Definitions of dimensionless variables
$ r_{\mathrm{D}}=\frac{r}{\alpha_2}$ $ t_{\mathrm{D}}=\frac{Q t}{2 \pi B \alpha_2^2 \theta_{2 \mathrm{~m}} R_{2 \mathrm{~m}}}$ $ C_{1 \mathrm{mD}}=\frac{C_{1 \mathrm{~m}}}{C_0}$ $ C_{1 \mathrm{imD}}=\frac{C_{1 \mathrm{im}}}{C_0}$ $ C_{2 \mathrm{mD}}=\frac{C_{2 \mathrm{mD}}}{C_0}$ $ C_{2 \mathrm{imD}}=\frac{C_{2 \mathrm{im}}}{C_0}$ $ \lambda_{1 \mathrm{mD}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q} \lambda_{1 \mathrm{~m}}$ $ \lambda_{2 \mathrm{mD}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q} \lambda_{2 \mathrm{~m}}$ $ \beta_2=\frac{\theta_{2 \mathrm{im}}}{\theta_{2 \mathrm{~m}}}$ $ \beta_1=\frac{\theta_{1 \mathrm{im}}}{\theta_{1 \mathrm{~m}}}$ $ \varepsilon_1=\frac{R_{1 \mathrm{~m}}}{R_{2 \mathrm{~m}}}$ $ \varepsilon_2=\frac{R_{1 \mathrm{im}}}{R_{2 \mathrm{~m}}}$ $ \varepsilon_3=\frac{R_{2 \mathrm{im}}}{R_{2 \mathrm{~m}}}$ $ \beta=\frac{V_{\mathrm{w}}}{2 \pi B \theta_{2 \mathrm{~m}} R_{2 \mathrm{~m}} \alpha_2^2}$ $ k_1=\frac{\alpha_1}{\alpha_2}$ $ k_2=\frac{\theta_{2 \mathrm{~m}}}{\theta_{1 \mathrm{~m}}}$ $ \omega_{1 \mathrm{D}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q \theta_{1 \mathrm{im}}} \omega_1$ $ \omega_{2 \mathrm{D}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q \theta_{2 \mathrm{im}}} \omega_2$ $ \lambda_{1 \mathrm{imD}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q} \lambda_{1 \mathrm{im}}$ $ \lambda_{2 \mathrm{imD}}=\frac{2 \pi B \theta_{2 \mathrm{~m}} \alpha_2^2}{Q} \lambda_{2 \mathrm{im}}$ 
下载: 导出CSV
表 2 模型参数默认取值
Table 2. Default parameter values used in this study
参数 值 参数 值 Q/(m3·h-1) 2.5 B/m 5 rw/m 0.1 r1/m 0.8 θ1m 0.15 θ2m 0.15 θ1im 0.1 θ2im 0.1 ω1/(1·h-1) 0.05 ω2/(1·h-1) 0.05 α1/m 0.5 α2/m 0.5
下载: 导出CSV
-
[1] Wang Y, Zheng C, Ma R. Safe and sustainable groundwater supply in China[J]. Hydrogeology Journal, 2018, 26(5): 1301-1324. doi: 10.1007/s10040-018-1795-1 [2] 王攀, 靳孟贵, 路东臣, 等. 永城市浅层地下水污染分布特征及来源识别[J]. 地质科技通报, 2022, 41(1): 260-268. doi: 10.19509/j.cnki.dzkq.2021.0136Wang P, Jin M G, Lu D C, et al. Distribution characteristics and source idenfication of shallow groundwater pollution in Yongcheng City[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 260-268(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2021.0136 [3] 肖骢. 变渗透性黏性土弱透水层中砷的迁移转化机制: 以江汉平原为例[D]. 武汉: 中国地质大学(武汉), 2019.Xiao C. Migration and transformation mechanism of arsenic in variable-permeability clayey aquitard at Jianghan Plain[D]. Wuhan: China University of Geosciences(Wuhan), 2019(in chinese with English abstract). [4] 江欣悦, 李静, 郭林, 等. 豫北平原浅层地下水化学特征与成因机制[J]. 地质科技通报, 2021, 40(5): 290-300. doi: 10.19509/j.cnki.dzkq.2021.0511Jiang X Y, Li J, Guo L, et al. Chemical characteristics and formation mechanism of shallow groundwater in the northern Henan Plain[J]. Bulletin of Geological Science and Technology, 2021, 40(5): 290-300(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2021.0511 [5] Hebig K H, Zeilfelder S, Ito N, et al. Study of the effects of the chaser in push-pull tracer tests by using temporal moment analysis[J]. Geothermics, 2015, 54: 43-53. doi: 10.1016/j.geothermics.2014.11.004 [6] 文章, 李旭. 考虑表皮效应的径向溶质迁移模型以及半解析解[J]. 地质科技通报, 2020, 39(1): 60-66. doi: 10.19509/j.cnki.dzkq.2020.0107Wen Z, Li X. Semi-analytical solution for radial solute transport model with skin effect[J]. Bulletin of Geological Science and Technology, 2020, 39(1): 60-66(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2020.0107 [7] Yeh H D, Chen Y J. Determination of skin and aquifer parameters for a slug test with wellbore-skin effect[J]. Journal of Hydrology, 2007, 342(3/4): 283-294. [8] 肖勋, 施文光, 王全荣. 井内混合效应与尺度效应对注入井附近溶质径向弥散过程的影响[J]. 地球科学, 2020, 45(4): 1439-1446. https://www.cnki.com.cn/Article/CJFDTOTAL-DQKX202004025.htmXiao X, Shi W G, Wang Q R. Effect of mixing effect and scale-dependent dispersion for radial solute transport near the injection well[J]. Earth Science, 2020, 45(4): 1439-1446(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DQKX202004025.htm [9] Huang J, Christ J A, Goltz M N. Analytical solutions for efficient interpretation of single-well push-pull tracer tests[J]. Water Resources Research, 2010, 46(8): LU08538. [10] Wang Q, Shi W, Zhan H, et al. Models of single-well push-pull test with mixing effect in the wellbore[J]. Water Resources Research, 2018, 54(12): 10155-160171. [11] De Hoog F R, Knight J, Stokes A. An improved method for numerical inversion of Laplace transforms[J]. SIAM Journal on Scientific and Statistical Computing, 1982, 3(3): 357-366. doi: 10.1137/0903022 [12] Schroth M H, Istok J D. Approximate solution for solute transport during spherical-flow push-pull tests[J]. Groundwater, 2005, 43(2): 280-284. doi: 10.1111/j.1745-6584.2005.0002.x [13] Chen K, Zhan H, Yang Q. Fractional models simulating non-Fickian behavior in four-stage single-well push-pull tests[J]. Water Resources Research, 2017, 53(11): 9528-9545. doi: 10.1002/2017WR021411 [14] Leij F J, Toride N, Field M S, et al. Solute transport in dual-permeability porous media[J]. Water Resources Research, 2012, 48(4): W04523. [15] 王宝辉, 董荟思, 徐兆明, 等. 多孔介质中污染物溶质迁移模型研究进展[J]. 化工进展, 2010, 29(7): 1338-1368. https://www.cnki.com.cn/Article/CJFDTOTAL-HGJZ201007033.htmWang B H, Dong H S, Xu Z M, et al. Research development in migration model of pollutant solute in porous media[J]. Chemical Industry and Engineering Progress, 2010, 29(7): 1338-1368(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-HGJZ201007033.htm [16] Van Genuchten M T, Wierenga P. Mass transfer studies in sorbing porous media I: Analytical solutions[J]. Soil Science Society of America Journal, 1976, 40(4): 473-480. [17] 高光耀, 冯绍元, 黄冠华. 饱和非均质土壤中溶质大尺度运移的两区模型模拟[J]. 土壤学报, 2008, 45(3): 398-404. https://www.cnki.com.cn/Article/CJFDTOTAL-TRXB200803002.htmGao G Y, Feng S Y, Huang G H. Simulation of solute transport at large scale in saturated heterogeneous soil with two-region model[J]. Acta Pedologica Sinica, 2008, 45(3): 398-404(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-TRXB200803002.htm [18] Gao G, Zhan H, Feng S, et al. A new mobile-immobile model for reactive solute transport with scale-dependent dispersion[J]. Water Resources Research, 2010, 46(8): W08533. [19] Zhou R, Zhan H, Chen K. Reactive solute transport in a filled single fracture-matrix system under unilateral and radial flows[J]. Advances in Water Resources, 2017, 104: 183-194. [20] Li N, Wen Z, Zhan H, et al. The single-well test dilemma: The skin effect and variable-rate pumping perspective[J]. Hydrogeology Journal, 2018, 26(7): 2521-2529. [21] Chen Y J, Yeh H D, Chang K J. A mathematical solution and analysis of contaminant transport in a radial two-zone confined aquifer[J]. Journal of Contaminant Hydrology, 2012, 138: 75-82. [22] 高光耀, 冯绍元, 马英, 等. 考虑弥散尺度效应的一维反应性溶质运移两区模型及应用[J]. 水利学报, 2011, 42(6): 631-640. https://www.cnki.com.cn/Article/CJFDTOTAL-SLXB201106004.htmGao G Y, Feng S Y, Ma Y, et al. One-dimensional two-region model for reactive solute transport with scale-dependent dispersion and its application[J]. Journal of Hydraulic Engineering, 2011, 42(6): 631-640(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-SLXB201106004.htm [23] Akter A. Rainwater harvesting: Building a water smart city[M]. Cham, Switzerland: Springer, 2022. [24] Wang Q, Wang J, Zhan H, et al. New model of reactive transport in a single-well push-pull test with aquitard effect and wellbore storage[J]. Hydrology and Earth System Sciences, 2020, 24(8): 3983-4000. [25] Li X, Wen Z, Zhu Q, et al. Numerical simulation of single-well push-pull tests in a radial two-zone confined aquifer[J]. Hydrogeology Journal, 2019, 27(7): 2645-2658. -
下载:
点击查看大图
计量
- 文章访问数: 588
- PDF下载量: 32
- 被引次数: 0
