A well flow model for a stratified heterogenous unconfined aquifer in a round island with infiltration recharge
-
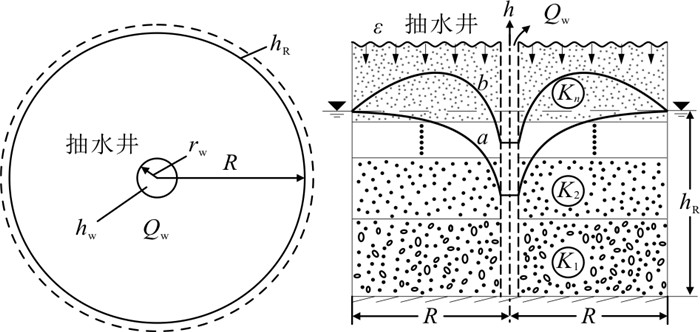
摘要:
作为圆岛状均质含水层稳定井流模型, 经典的Dupuit井流模型既没有考虑普遍存在的降水入渗补给, 也不适用于层状非均质含水层系统, 有必要加以完善。在考虑降水入渗补给改进Dupuit井流模型的基础上, 进一步将其拓展到层状非均质潜水含水层。引入Гиринский(吉林斯基)势函数, 根据水均衡原理建立极坐标下的地下水流微分方程, 再依边界条件解析得到了相应的流量方程、水位方程和分水岭公式。以双层结构为例, 观察30组不同参数条件的典型水位曲线组, 发现不同渗透系数的水位曲线交于一点的特殊现象并从理论上给出了证明。解析模型仍引入Dupuit假定, 且没有考虑抽水井的井壁"水跃"现象, 为判断这些条件对解析公式适用能力的影响, 建立轴对称剖面二维流数值模型并做了对比研究。除抽水井附近外, 水位解析方程产生的相对误差一般低于4%, 在最偏离Dupuit假定的分水岭处, 距离和水位的解析误差均小于0.1%。Dupuit假定并没有严重影响解析模型的适用性。
Abstract:Objective The Dupuit model of well flow is a classical steady-state well flow model for a homogeneous unconfined aquifer in a round island. However, it does not consider the widely existing infiltration recharge from precipitation, and is also inapplicable for stratified heterogeneous aquifer systems. Therefore, it must be modified to address these issues.
Methods On the basis of the revised Dupuit well flow model which incorporates infiltration recharge, this study further extended its application to a stratified heterogeneous unconfined aquifer. The Girinskii's potential function was used to construct the differential equations for the radial groundwater flow according to the water balance principle, and the analytical solutions satisfying the boundary conditions are then obtained as formulas of the flow rate, water table and groundwater divide. Taking the bilayer structure as an example, typical groundwater level curves with respect to 30 scenarios of different parameter values were investigated. A special phenomenon was found in which the curves of different hydraulic conductivities intersect at a single point, which could also be proven in theory. This analytical model still adopted the Dupuit assumption and did not consider the "hydraulic jump" phenomenon on the wall of the pumping well. To check the impact of these constraints on the applicability of the analytical formulas, a two-dimensional numerical model for axially symmetric seepage was built for comparison.
Results As indicated by the results, the relative error of the groundwater level estimated from the analytical solution is generally less than 4%, except for the zone near the pumping well. On the groundwater divide, where the Dupuit assumption is mostly invalid, the relative errors of the analytical solution to both the distance and height of the divide are smaller than 0.1%.
Conclusion The Dupuit assumption does not significantly influence applicability of the analytical model.
-
图 1 层状非均质含水层具入渗补给的圆岛状潜水井流模型图
K0, K1, K2, …, Kn分别为第1, 第2, …, 第n介质层渗透系数(m/d)；Qw为抽水流量(m3/d)；hR为圆岛外边界水位(m)；hw为开采井中水位(m)；R为圆岛半径(m)；a为无分水岭水位曲线；b为有分水岭水位曲线
Figure 1. Model map of well flow in a round island with a stratified heterogeneous unconfined aquifer obtaining infiltration recharge
图 2 层状非均质含水层吉林斯基势函数定义要素图
z1, z2, …, zn分别为抽水井所揭露第1, 第2, …, 第n介质层底部标高(m)；M1, M2, …, Mn分别为第1, 第2, …, 第n介质层的水带厚度(m)
Figure 2. Elements of Girinskii's potential function for a stratified heterogeneous aquifer
图 3 双层结构含水层天然状态水位曲线计算案例(ε.入渗强度；K2.水平渗透系数; 下同)
Figure 3. Estimated results of groundwater level curves in the natural state for an aquifer of the bilayer structure
图 4 双层结构含水层在Qw=5 000 m3/d抽水状态水位曲线计算案例
Figure 4. Estimated results of groundwater level curves in the pumping state of Qw=5 000 m3/d for an aquifer of the bilayer structure
图 5 解释交叉点的f(r/R)函数曲线
Figure 5. Function curve of f(r/R) used to interpret the intersection poi
图 6 双层结构含水层轴对称剖面二维流概念模型示意图
Figure 6. Schematic diagram of two-dimensional flow conceptual model in axisymmetric section of bilayer structure aquifer
图 8 抽水井附近解析水位误差随距离的变化曲线图
Figure 8. Relationship between the error of the analytical groundwater level and the distance near the pumping well
图 9 分水岭处解析水位误差随K2的变化曲线图
Figure 9. Relationship between the error of the analytical groundwater level and the K2 value on the groundwater divide
表 1 Qw=0 m3/d时不同K2值r=0处水位峰值hp
Table 1. Maximum groundwater level, hp at r=0 for different K2 values when Qw=0 m3/d
ε/(m·d-1) 水位峰值hp/m K2=1 K2=5 K2=10 K2=20 K2=100 0.000 75 52.34 51.85 51.48 51.06 50.32 0.001 53.11 52.46 51.96 51.40 50.43 0.001 5 54.65 53.67 52.92 52.08 50.64 注：K2单位为m/d 
下载: 导出CSV
表 2 Qw=5 000 m3/d时不同K2值抽水井的井中水位hw
Table 2. Water level within the pumping well for different K2 values when Qw=5 000 m3/d
ε/(m·d-1) 井中水位hw/m K2=1 K2=5 K2=10 K2=20 K2=100 0.000 75 26.72 29.56 32.77 37.63 46.66 0.001 27.64 30.40 33.52 38.17 46.78 0.001 5 29.39 32.03 34.98 39.23 47.03 注：K2单位为m/d
下载: 导出CSV
表 3 Qw=5 000 m3/d时分水岭和交叉点特征
Table 3. Characteristics of groundwater divide and intersection point for Qw=5 000 m3/d
ε/(m·d-1) 分水岭位置rd/m 不同K2值(m/d)对应分水岭处水位hd(m) 交叉点位置rc/m 交叉点水位hc/m K2=1 K2=5 K2=10 K2=20 K2=100 0.000 75 1 456.732 50.31 50.25 50.20 50.14 50.04 974.95 50.000 0.001 1 261.567 50.73 50.59 50.47 50.33 50.10 650.02 50.000 0.0015 1 030.065 51.79 51.42 51.14 50.81 50.25 318.55 50.000 注：K2单位为m/d
下载: 导出CSV
-
[1] Dupuit AJE J. Etudes theoretiques et pratiques sur le mouvement des eaux[M]. Paris: Dunod, 1863. [2] 卡明斯基. 地下水动力学原理[M]. 北京地质学院水文地质教研室, 译. 北京: 地质出版社, 1955.Каменский Г И. Основы Дина-мики Подземных Вод[M]. Госгеолиздат: Москва, 1943 (in Russian). [3] 贝尔. 多孔介质流体动力学[M]. 李竞生, 陈崇希, 译. 北京: 中国建筑工业出版社, 1983.Bear J. Dynamics of fluids in porous media[M]. New York: Elsevier, 1972. [4] 陈崇希. 地下水不稳定井流计算方法[M]. 北京: 地质出版社, 1983.Chen C X. Calculation method of unsteady well flow of groundwater[M]. Beijing: Geological Publishing House, 1983 (in Chinese). [5] 陈崇希, 林敏, 成建梅. 地下水动力学: 第5版[M]. 北京: 地质出版社, 2011.Chen C X, Lin M, Cheng J M. Groundwater dynamics: 5th Edition[M]. Beijing: Geological Publishing House, 2011(in Chinese). [6] 陈崇希. Dupuit模型的改进: 具入渗补给[J]. 水文地质工程地质, 2020, 47(5): 1-4.Chen C X. Improvement of Dupuit model: With infiltration recharge[J]. Hydrogeology & Engineering Geology, 2020, 47(5): 1-4(in Chinese with English abstract). [7] 吉林斯基. 渗透系数测定法[M]. 冉造, 译. 北京: 地质出版社, 1958.Гиринский Н К. При Неуcтaновившихся Дeвитe И Понижениях[M]. Гоcгeолиздат: Москва, 1950 (in Russian). [8] 阿拉文, 奴米罗夫. 水工建筑物的渗透计算[M]. 伍修焘, 李协生, 译. 北京: 水利电力出版社, 1959.Аравин В N, Hумеров С Н. Филътрационные Расчеты Гидротехничеких Сооружений[M]. СТР И АРХ: Ленинград, 1955 (in Russian). [9] Todd D K. Ground Water Hydrology[M]. New York: John Wiley @ Sons, Inc, 1959. [10] Haitjema H M. Analytic Element Modeling of Groundwater Flow[M]. San Diego: Academic Press, Inc., 1995. [11] Kitterød N O. Dupuit-Forchheimer solutions for radial flow with linearly varying hydraulic conductivity or thickness of aquifer[J]. Water Resources Research, 2004, 40(11): W11507. [12] 陈崇希. 地下水动力学[M]. 北京: 北京地质学院, 1966.Chen C X. Groundwater hydraulics[M]. Beijing: Beijing Institute of Geology, 1966(in Chinese). [13] Theis C V. The relation between the lowering of the piezometric surface and the rate and duration of discharge of a well using groundwater storage[J]. Trans. Amer. Geophys. Union, 1935, 16: 519-524. doi: 10.1029/TR016i002p00519 [14] McDonald M G, Harbaugh A W. A modular three-dimensional finite-difference ground-water flow model[R]. USGS: Techniques of Water-Resources Investigations of the U.S. Geological Survey, Chapter A1, Book 6, 1988: 3-191. [15] 王旭升. 潜水稳定井流问题剖面二维渗流的等效数值模拟[J]. 地质科技通报, 2023, 42(4): 27-36. doi: 10.19509/j.cnki.dzkq.tb20230024Wang X S. Equivalent two-dimensional numerical modeling of well flow in an unconfined aquifer[J]. Bulletin of Geological Science and Technology, 2023, 42(4): 27-36(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.tb20230024 [16] 陈崇希, 唐仲华. Theis不稳定潜水井流模型的改进: 具入渗补给[J]. 水文地质工程地质, 2021, 48(6): 1-12.Chen C X, Tang Z H. Improvement of the Theis unsteady well flow model with infiltration recharge in a phreatic aquifer[J]. Hydrogeology & Engineering Geology, 2021, 48(6): 1-12(in Chinese with English abstract). [17] 陈崇希, 林敏, 叶善士, 等. 地下水混合井流的理论及应用[M]. 武汉: 中国地质大学出版社, 1998.Chen C X, Lin M, Ye S S, et al. Theory of multi-layer mixed well flow and its application[M]. Wuhan: China University of Geosciences Press, 1998(in Chinese). [18] 陈崇希, 蒋健民, 林敏, 等. 地下水不稳定混合抽水的渗流-管流耦合模型及其应用[R]. 南宁: 广西地质矿产局, 1993: 1-199.Chen C X, Jiang J M, Lin M, et al. Coupling model of seepage and pipe flow for multi-layer mixed unsteady groundwater flow to pumping wells[R]. Nanning: Guangxi Bureau of Geology and Mineral Resources, 1993: 1-199(in Chinese). [19] 陈崇希. 地下水不稳定混合抽水的模型及模拟方法[J]. 地球学报, 1996, 17(增刊): 222-228. https://cpfd.cnki.com.cn/Article/CPFDTOTAL-DQXB199612001033.htmChen C X. The model and simulated method for the mix-pumping unstable ground water[J]. Acta Geoscientia Sinica, 1996, 17(S): 222-228(in Chinese with English abstract). https://cpfd.cnki.com.cn/Article/CPFDTOTAL-DQXB199612001033.htm [20] Chen C X, Jiao J J. Numerical simulation of pumping test in multilayer wells with non-Darcian flow in the wellbore[J]. Ground Water, 1999, 37(3): 465-474. doi: 10.1111/j.1745-6584.1999.tb01126.x [21] 陈崇希. 岩溶管道-裂隙-空隙三重空隙介质地下水流模型及模拟方法研究[J]. 地球科学, 1995, 20(4): 361-366.Chen C X. Groundwater flow model and simulation method in triple media of Karstic tube-fissure-pore[J]. Earth Science: Journal of China University of Geosciences, 1995, 20(4): 361-366(in Chinese with English abstract). [22] 陈崇希. 三维地下水流中常规观测孔水位的形成机理及确定方法[J]. 地球科学, 2003, 28(5): 483-492. https://www.cnki.com.cn/Article/CJFDTOTAL-DQKX200305002.htmChen C X. Formation mechanism of water level and its determination method in conventional observation wells for Three-Dimensional groundwater flow[J]. Earth Science: Journal of China University of Geosciences, 2003, 28(5): 483-492(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DQKX200305002.htm [23] Chen C X, Wan J W, Zhan H B. Theoretical and experimental studies of coupled seepage-pipe flow to a horizontal well[J]. Journal of Hydrology, 2003, 281(1/2): 163-175. -
下载:
点击查看大图
计量
- 文章访问数: 481
- PDF下载量: 86
- 被引次数: 0
