Study on the upscaling transformation of hydraulic conductivity in fractured media
-
摘要:
研究裂隙介质渗透性的升尺度转换, 对准确刻画裂隙介质渗流场特征具有非常重要的意义。以某地下水封洞库的结晶岩裂隙介质统计数据为基础, 应用Monte-Carlo随机模拟技术生成二维离散裂隙网络(DFN)模型, 计算得到变尺寸模拟域的渗透性参数及不同尺寸网格化划分后各网格单元的等效渗透系数, 并对网格单元等效渗透系数进行升尺度运算。结果表明: 模拟域尺寸达到渗透性典型单元体(REV)尺寸22 m×22 m后, 模拟域可视为等效连续介质; 网格化处理后, 小于REV尺寸的网格单元升尺度运算得到的等效渗透系数显著小于裂隙网络模型计算得到的对应复合网格单元的等效渗透系数。因此, 渗流计算单元尺寸达到REV尺寸后, 其渗透性参数可以有效代表研究区内更大尺寸区域的渗透性特征; 当渗流计算单元尺寸小于REV尺寸时, 其渗透性参数无法有效代表研究区内更大尺寸区域的渗透性特征, 此时对渗透性参数进行参数升尺度运算往往具有低估的误差, 不具有实际意义。
Abstract:Objective It is very important to study the upscaling transformation of hydraulic conductivity in fracture media for accurately characterizing the seepage field characteristics.
Methods Based on the fracture medium statistics of crystalline rock in an underground water-sealed cavern, a 2-dimensional discrete fracture network (DFN) model is generated using the Monte-Carlo stochastic simulation technique. The hydraulic conductivity parameters of the variable size simulation domain and the equivalent hydraulic conductivity of each grid element after meshing in different sizes are calculated. The hydraulic conductivity representative elementary volume (REV) of the study area was analysed by the variation in hydraulic conductivity parameters with the size of the simulation area, and the equivalent hydraulic conductivity of grid cells smaller than REV was calculated by upscaling.
Results The results show that the simulation domain can be regarded as an equivalent continuum when the size of the REV reaches 22 m×22 m. After meshing treatment, the equivalent hydraulic conductivity of the mesh cells which is smaller than REV calculated by the upscaling operation is significantly smaller than that of the corresponding composite mesh cells calculated by the crack network model.
Conclusion Therefore, when the size of the seepage calculation unit reaches the REV size, its hydraulic conductivity parameters can effectively represent the hydraulic conductivity characteristics of a larger area in the study area. However, when the size of the seepage calculation unit is smaller than the REV size, its hydraulic conductivity parameters cannot effectively represent the hydraulic conductivity characteristics of the larger area in the study area. As a consequence, parameter upscaling calculation on the hydraulic conductivity parameters often has underestimated errors and is not of practical significance. For fractured media study areas with insufficient data, it is often difficult to determine the hydraulic conductivity REV of the study area. In this case, it can be considered that there is usually an underestimation error when the hydraulic conductivity of the small-scale area obtained from the hydrogeological test is upscaled. This conclusion provides a theoretical basis for the numerical simulation of the seepage field in fracture media in various related engineering projects.
-
Key words:
- discrete fracture network model /
- upscaling /
- REV /
- hydraulic conductivity tensor /
- fractured media
-
图 1 优势结构面1，2，3方向角分布直方图
Figure 1. Histogram of the angular distribution in directions 1, 2 and 3 of the dominant structural plane
图 2 优势结构面1，2，3迹长分布直方图
Figure 2. Histogram of trace length distribution of dominant structural planes 1, 2 and 3
图 3 基于烟台洞库数据的二维裂隙网络模型效果图
Figure 3. Effect of the two-dimensional fracture network model based on Yantai cave data
图 4 裂隙网络模型绕中心点旋转示意图
Figure 4. Diagram of the fracture network model rotating around the center point
图 5 裂隙网络模型渗透椭圆示意图
Figure 5. Hydraulic conductivity ellipse diagram of the fracture network model
图 6 渗透张量分量随模拟域尺寸变化图
Figure 6. Hydraulic conductivity tensor component changes with the size of the simulation domain
图 8 变异系数随模拟域尺寸变化图
Figure 8. Coefficient of variation with the size of the simulation domain
图 9 二维标准重整化过程示意图
Figure 9. Schematic diagram of a two-dimensional standard renormalization process
图 10 二维简化重整化过程示意图
Figure 10. Schematic diagram of a two-dimensional simplified renormalization process
图 11 m网格单元等效渗透系数升尺度展示图
Figure 11. Upscaling diagram of the equivalent permeability coefficient of a 5 m grid cell
图 12 10 m网格单元等效渗透系数升尺度展示图
Figure 12. Upscaling diagram of the equivalent permeability coefficient of a 10 m grid cell
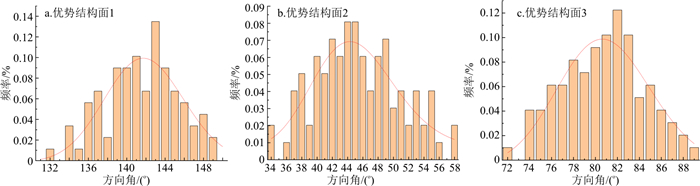
表 1 优势裂隙组各个几何参数分布类型及统计规律
Table 1. Distribution types and statistical rules of geometric parameters in the dominant fracture group
优势裂隙组数 方向角(正态分布) 迹长(对数正态分布) 隙宽(对数正态分布) 密度/(条·m-2) 均值/(°) 标准差/(°) 均值/m 标准差/m 均值/m 标准差/m 1 142.29 3.78 5.23 2.90 0.000 4 0.000 2 0.29 2 45.91 5.51 5.62 2.67 0.000 4 0.000 2 0.23 3 81.29 3.63 6.42 3.34 0.000 4 0.000 2 0.24 
下载: 导出CSV
-
[1] 贾群龙, 邢立亭, 于苗, 等. 裂隙岩溶介质渗透性变异规律的尺度效应[J]. 干旱区资源与环境, 2022, 36(12): 127-134. https://www.cnki.com.cn/Article/CJFDTOTAL-GHZH202212017.htmJia Q L, Xing L T, Yu M, et al. Scale effect of permeability variation pattern of fracture-karst media[J]. Journal of Arid Land Resources and Environment, 2022, 36(12): 127-134(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-GHZH202212017.htm [2] 陈刚. 基于多尺度三维空间裂隙分布的粗糙岩体裂隙渗透性研究[D]. 昆明: 昆明理工大学, 2021.Chen G. Fracture permeability of rough rock mass based on multi-scale three-dimensional spatial fracture distribution[D]. Kunming: Kunming University of Science and Technology, 2021(in Chinese with English abstract). [3] 孙蓉琳, 梁杏, 靳孟贵. 裂隙岩体渗透系数确定方法综述[J]. 水文地质工程地质, 2006, 33(6): 120-123. doi: 10.3969/j.issn.1000-3665.2006.06.030Sun R L, Liang X, Qin M G. Review on determination of hydraulic conductivity of fractured rocks[J]. Hydrogeology & Engineering Geology, 2006, 33(6): 120-123(in Chinese with English abstract). doi: 10.3969/j.issn.1000-3665.2006.06.030 [4] 刘日成, 蒋宇静, 李博, 等. 岩体裂隙网络等效渗透系数方向性的数值计算[J]. 岩土力学, 2014, 35(8): 2394-2400. doi: 10.16285/j.rsm.2014.08.016Liu R C, Jiang Y J, Li B, et al. Numerical calculation of directivity of equivalent permeability of fractured rock masses network[J]. Rock and Soil Mechanics, 2014, 35(8): 2394-2400(in Chinese with English abstract). doi: 10.16285/j.rsm.2014.08.016 [5] 高超, 钟振, 胡云进, 等. 岩体单裂隙渗透性尺寸效应的数值模拟研究[J]. 水利水电技术, 2018, 49(12): 151-156. https://www.cnki.com.cn/Article/CJFDTOTAL-SJWJ201812020.htmGao C, Zhong Z, Hu Y J, et al. Numerical simulation on size effect of single rock fracture permeability[J]. Water Resources and Hydropower Engineering, 2018, 49(12): 151-156(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-SJWJ201812020.htm [6] Rukaviková L, Holeek J, Holeková P, et al. Comparison of hydraulic conductivity of rock matrix and fractured blocks of granitic rocks[J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 144: 104743. doi: 10.1016/j.ijrmms.2021.104743 [7] Dentz M, Le Borgne T, Englert A, et al. Mixing, spreading and reaction in heterogeneous media: A brief review[J]. Journal of Contaminant Hydrology, 2011, 120/121(S1): 1-17. [8] Renard P, Le Loc'h G, Ledoux E, et al. A fast algorithm for the estimation of the equivalent hydraulic conductivity of heterogeneous media[J]. Water Resources Research, 2000, 36(12): 3567-3580. doi: 10.1029/2000WR900203 [9] Dagan G, Fiori A, Jankovic I. Upscaling of flow in heterogeneous porous formations: Critical examination and issues of principle[J]. Advances in Water Resources, 2013, 51: 67-85. doi: 10.1016/j.advwatres.2011.12.017 [10] 覃荣高, 曹广祝, 仵彦卿. 非均质含水层中渗流与溶质运移研究进展[J]. 地球科学进展, 2014, 29(1): 30-41. https://www.cnki.com.cn/Article/CJFDTOTAL-DXJZ201401004.htmQin R G, Cao G Z, Wu Y Q. Review of the study of groundwater flow and solute transport in heterogeneous aquifer[J]. Advances in Earth Science, 2014, 29(1): 30-41(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DXJZ201401004.htm [11] 郭富欣, 王亚青, 尚凡杰, 等. 渗透率粗化方法对比与优选: 以巴西Libra油田为例[J]. 大庆石油地质与开发, 2019, 38(2): 58-66. https://www.cnki.com.cn/Article/CJFDTOTAL-DQSK201902009.htmGuo F X, Wang Y Q, Shang F J, et al. Comparison and optimization of the permeability upscaling methods: A case study on Libra Oilfield in Brazil[J]. Petroleum Geology & Oilfield Development in Daqing, 2019, 38(2): 58-66(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DQSK201902009.htm [12] 胡成, 陈刚, 曹孟雄, 等. 基于离散裂隙网络法和水流数值模拟技术的地下水封洞库水封性研究[J]. 地质科技通报, 2022, 41(1): 119-126, 136. doi: 10.19509/j.cnki.dzkq.2022.0029Hu C, Chen G, Cao M X, et al. A case study on water sealing efficieny of groundwater storage caverns using discrete fracture network method and flow numerical simulation[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 119-126, 136(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0029 [13] Gong B, Karimi-Fard M, Durlofsky L J. Upscaling discrete fracture characterizations to dual-porosity, dual-permeability models for efficient simulation of flow with strong gravitational effects[J]. Spe Journal, 2008, 13(1): 58-67. [14] Fiori A, Dagan G, Jankovic I. Upscaling of steady flow in three dimensional highly heterogeneous formation[J]. Multiscale Modeling & Simulation, 2011, 9(3): 1162-1180. [15] Li J C, Lei Z D, Qin G, et al. Effective local-global upscaling of fractured reservoirs under discrete fractured discretization[J]. Energies, 2015, 8(9): 10178-10197. [16] 宛东, 胡成, 邢文乐. 离散裂隙网络模型在矿井涌水量预测中的应用[J]. 河北地质大学学报, 2018, 41(1): 65-69. https://www.cnki.com.cn/Article/CJFDTOTAL-HBDX201801007.htmWan D, Hu C, Xing W L. Application of discrete fracture network model to mine water inflow prediction[J]. Journal of Hebei GEO University, 2018, 41(1): 65-69(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-HBDX201801007.htm [17] 刘恒伟, 肖鹏, 窦斌, 等. 储层特征对水平井多裂隙增强型地热系统采热过程影响的数值模拟研究[J]. 地质科技通报, 2022, 41(3): 341-348. doi: 10.19509/j.cnki.dzkq.2022.0081Liu H W, Xiao P, Dou B, et al. Numerical simulation of influence of reservoir characteristics on heating process of enhanced geothermal system of horizontal well multifractures[J]. Bulletin of Geological Science and Technology, 2022, 41(3): 341-348(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0081 [18] Ma G W, Li T, Wang Y, et al. The equivalent discrete fracture networks based on the correlation index in highly fractured rock masses[J]. Engineering Geology, 2019, 260: 105228. [19] Zhu X M, Liu G N, Gao F, et al. A complex network model for analysis of fractured rock permeability[J]. Advances in Civil Engineering, 2020, 2020: 8824082. [20] Öhman J, Niemi A. Upscaling of fracture hydraulics by means of an oriented correlated stochastic continuum model[J]. Water Resources Research, 2003, 39(10): 1277. [21] Min K B, Jing L R. Numerical determination of the equivalent elastic compliance tensor for fractured rock masses using the distinct element method[J]. International Journal of Rock Mechanics & Mining Sciences, 2003, 40(6): 795-816. [22] 张弛. 结合升尺度方法的地下水污染物迁移研究[D]. 上海: 上海交通大学, 2014.Zhang C. Numerical modeling of groundwater contaminant transport with upscaling methods[D]. Shanghai: Shanghai Jiao Tong University, 2014(in Chinese with English abstract). [23] King P R. The use of renormalization for calculating effective permeability[J]. Transport in Porous Media, 1989, 4(1): 37-58. [24] Renard P, Le Loc'h G, Ledoux E, et al. A fast algorithm for the estimation of the equivalent hydraulic conductivity of heterogeneous media[J]. Water Resources Research, 2000, 36(12): 3567-3580. [25] 黎照洪, 胡成, 陈刚, 等. 基于离散裂隙网络的烟台水封洞库渗水点分析[J]. 安全与环境工程, 2016, 23(5): 170-173, 182. https://www.cnki.com.cn/Article/CJFDTOTAL-KTAQ201605031.htmLi Z H, Hu C, Chen G, et al. Seepage analysis of the water-sealed cavern in yantai city based on the discrete fracture network model[J]. Safety and Environmental Engineering, 2016, 23(5): 170-173, 182(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-KTAQ201605031.htm -
下载:
点击查看大图
计量
- 文章访问数: 1057
- PDF下载量: 61
- 被引次数: 0
