潜水稳定井流的剖面二维数值模拟方法

王旭升; 谢永桦; 陈崇希

doi:10.19509/j.cnki.dzkq.tb20230024

潜水稳定井流的剖面二维数值模拟方法

doi: 10.19509/j.cnki.dzkq.tb20230024

1a.
中国地质大学(北京)水利部地下水保护重点实验室(筹), 北京 100083
1b.
中国地质大学(北京)水资源与环境学院, 北京 100083
2.
中国地质大学(武汉)环境学院, 武汉 430078

基金项目:

国家自然科学基金项目 41972263

国家自然科学基金项目 41772249

详细信息

作者简介:
王旭升(1974—), 男, 教授, 博士生导师, 主要从事地下水动力学和水文模型研究工作。E-mail: wxsh@cugb.edu.cn

中图分类号: P641
计量
- 文章访问数: 859
- PDF下载量: 84
- 被引次数: 0
出版历程
- 收稿日期: 2023-01-13
- 录用日期: 2023-04-19
- 修回日期: 2023-04-14

Sectional 2D numerical modelling method for steady state well-flow in an unconfined aquifer

1a.
Key Laboratory of Groundwater Conservation of Ministry of Water Resources(in preparation), China University of Geosciences(Beijing), Beijing 100083, China
1b.
School of Water Resources & Environment, China University of Geosciences(Beijing), Beijing 100083, China
2.
School of Environment Studies, China University of Geosciences(Wuhan), Wuhan 430078, China

摘要

摘要:
经典Dupuit井流模型与考虑入渗的改进Dupuit井流模型, 都受到Dupuit假定的影响, 可能存在系统误差。建立反映三维流或轴对称二维流特性的潜水井流数值模型, 是检验Dupuit模型可靠性的重要手段。提出一种模拟潜水稳定井流的剖面二维数值模拟方法, 通过参数转换把柱坐标系的渗流方程变换为等效的直角坐标系方程, 利用MODFLOW方体网格有限差分模型实现剖面二维流场模拟。数值模型以抽水井的井中水位为已知条件, 渗出面的排水量按照Darcy定律差分公式计算, 潜水面则通过MODFLOW对干-湿单元的处理加以识别, 抽水流量经水均衡计算得到。通过采用精细化网格建立典型案例模型, 获得模拟精度很高的结果, 使反算抽水井流量的相对误差不超过0.2%。以此检验Dupuit井流模型, 发现解析公式得到的水位线总体与数值模拟结果一致, 仅在抽水井附近由于没有考虑水跃而偏低, 且误差受到含水层渗透系数各向异性的影响。在有入渗的情况下, 分水岭附近的渗流违反Dupuit假定。然而, 改进的Dupuit井流公式计算的分水岭水位相对误差低于1%。这一数值模拟方法简单实用, 但也受到MODFLOW本身局限性的约束。
- Dupuit假定 /
- 抽水井 /
- 入渗补给 /
- 渗出面 /
- 潜水面 /
- 各向异性 /
- 有限差分法
Abstract: Objective
Both the classical Dupuit model and the modified Dupuit model including infiltration are influenced by Dupuit's assumption and have potential systematic errors. Building a numerical model of well flow in an unconfined aquifer by characterizing the three-dimensional or axisymmetric two-dimensional (2D) flow is an essential approach to verify the performance of Dupuit-type models.
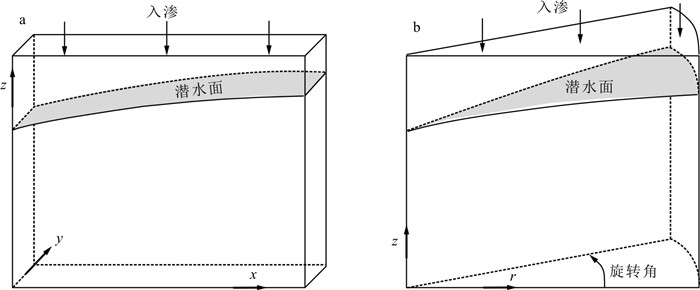
Methods
In this study, a 2D numerical model is proposed for the steady state well-flow in an unconfined aquifer, in which the control equation of seepage in the cylindrical coordinates is transformed equivalently to the Cartesian coordinates through parameter transformation, and the sectional 2D modelling is implemented via the MODFLOW finite-difference grid of cubic blocks. In the numeric model, the water level in the pumping well is a given condition, the flux across the seepage face is estimated by difference equation according to Darcy's law, the phreatic surface is identified by the treatment of dry and wet cells in MODFLOW, and the pumping rate is determined from the water debug calculation.
Results
Fine grids are constructed innumerical models of typical cases to obtain high-precision results, in which the relative error of the backwards estimated pumping rate is no more than 0.2%. This numerical model is used to check the Dupuit-type well-flow models. As indicated, the groundwater level estimated from the analytical formulas generally agrees well with the numerical modelling results, except that near the well, where the analytical solution underestimates the groundwater level due to ignoring the waterjump and the errors depend on the anisotropic permeability of the aquifer. When infiltration exists, the flow in the vicinity of watershed does not follow Dupuit's assumption. However, the estimated groundwater level on the watershed by modified Dupuit well-flow equation has a low level of relative error, which is less than 1%.
Conclusion
This numerical method is simple and practical however it is also influenced by limitations in MODFLOW.
- Dupuit's assumption /
- pumping well /
- infiltration recharge /
- seepage face /
- phreetic surface /
- anisotropic /
- finite difference method
注释:

(所有作者声明不存在利益冲突)

HTML全文

图 1 直角坐标系(a)和柱坐标系(b)的潜水面示意图(z为相对高度; x为横向距离; r为径向距离；后同)

Figure 1. Schematics of the phreetic surface in the cartesian (a) and cylindrical (b) coordinate systems

下载: 全尺寸图片幻灯片

图 2 含水层网格单元与井孔的关系示意图

a.井壁渗出面相邻单元；b.井孔饱水带相邻单元；r_w为抽水井半径；Δx为单元长度；Δz为单元厚度；Δy为单元宽度；Q_x为侧面流量；z_w为井中水位；z(k)为单元中点高度；H(k)为单元格点水头

Figure 2. Schematics of the relationship between grid blocks of the aquifer and the well

下载: 全尺寸图片幻灯片

图 3 潜水井流模型示意图

x, r为距离；R为圆岛半径；L_R为外侧边界水跃；H(r, z)为水头分布函数；h_w为井中水位；L_w为井壁水跃

Figure 3. Diagrammatic map of the model for the wellflow in an unconfined aquifer

下载: 全尺寸图片幻灯片

图 4 情景A模拟水位与经典Dupuit模型解析解的局部对比

Figure 4. Local comparison between the modelled groundwater level of the scenario-A and the analytical solution of the classical Dupuit model

下载: 全尺寸图片幻灯片

图 5 情景B模拟水位与改进Dupuit模型解析解的对比

a.整体范围；b.抽水井附近

Figure 5. Comparison between the modelled groundwater level of scenario-B and the analytical solution of the modified Dupuit model

下载: 全尺寸图片幻灯片

图 6 情景C的模拟水位与改进Dupuit模型解析解的对比

a.抽水井附近；b.全部范围

Figure 6. Comparison between the modelled groundwater level of scenario-C and the analytical solution of the modified Dupuit model

下载: 全尺寸图片幻灯片

图 7 情景D的模拟结果

Figure 7. Numerical modelling results of scenario-D

下载: 全尺寸图片幻灯片

表 1 模拟情景编号和参数

Table 1. Code and parameters of modelling scenarios

模拟情景	水平方向渗透系数K_h/(m·d^-1)	各向异性比值K_v/K_h	入渗补给强度ε/(mm·d^-1)	井中水位h_w/m
A	10	1	0.0	35
B	10	1	1.0	35
C	10	0.1, 10.0	1.0	35
D	1	0.1	1.0	20

下载: 导出CSV

参考文献(25)

[1]	Dupuit A J E J. Etudes theoretiques et pratiques sur le mouvement des eaux[M]. Paris: Dunod, 1863.
[2]	Каменский Г И. Основы дина-мики подземных вод[M]. Москва: Госгеолиздат, 1943(in Russian).
[3]	Haitjema H M. Analytic element modeling of groundwater flow[M]. San Diego: Academic Press, Inc., 1995.
[4]	陈崇希. Dupuit模型的改进: 具入渗补给[J]. 水文地质工程地质, 2020, 47(5): 1-4. https://www.cnki.com.cn/Article/CJFDTOTAL-SWDG202106001.htm Chen C X. Improvement of Dupuit model: With infiltration recharge[J]. Hydrogeology & Engineering Geology, 2020, 47(5): 1-4(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-SWDG202106001.htm
[5]	Чарный И A. Строгое доказателъство формулм дюпюидля безнапорной филътрации с промеҗутком высачнвания[J]. Докл. АН СССР, 1951, 79(6): 937-948. https://www.cnki.com.cn/Article/CJFDTOTAL-NWYJ202105015.htm
[6]	陈崇希, 林敏. 地下水动力学[M]. 武汉: 中国地质大学出版社, 1999. Chen C X, Lin M. Groundwater hydraulics[M]. Wuhan: China University of Geosciences Press, 1999(in Chinese).
[7]	Boulton N S. The flow pattern near a gravity well in a uniform water-bearing medium[J]. Journal of the ICE, 1951, 36(10): 534-550.
[8]	张有龄. 河床地下水运动的供水理论分析[M]. 北京: 科学出版社, 1958. Zhang Y L. Theoretical analysis on water yield from groundwater flow beneath riverbed[M]. Beijing: Science Press, 1958(in Chinese).
[9]	Taylor G S, Luthin J N. Computer methods for transient analysis of water-table aquifers[J]. Water Resources Research, 1969, 5(1): 144-152. doi: 10.1029/WR005i001p00144
[10]	Neuman S P, Witherspoon P A. Finite element method of analyzing steady seepage with a free surface[J]. Water Resources Research, 1970, 6(3): 889-897. doi: 10.1029/WR006i003p00889
[11]	McDonald M G, Harbaugh A W. A modular three-dimensional finite-difference ground-water flow model[R]. Denver: Techniques of Water-Resources Investigations of the U.S. Geological Survey, Chapter A1, Book 6, 1988.
[12]	王旭升, 万力. 地下水运动方程[M]. 北京: 地质出版社, 2011. Wang X S, Wan L. Equations of groundwater movements[M]. Beijing: Geological Publishing House, 2011(in Chinese).
[13]	陈崇希, 林敏, 成建梅. 地下水动力学[M]. 北京: 地质出版社, 2011. Chen C X, Lin M, Cheng J M. Groundwater hydraulics[M]. Beijing: Geological Publishing House, 2011(in Chinese).
[14]	Samani N, Kompani-Zarea M, Barry D. MODFLOW equipped with a new method for the accurate simulation of axisymmetric flow[J]. Advances in Water Resources, 2004, 27(1): 31-45. doi: 10.1016/j.advwatres.2003.09.005
[15]	Langevin C D. Modeling axisymmetric flow and transport[J]. Groundwater, 2008, 46(4): 579-590. doi: 10.1111/j.1745-6584.2008.00445.x
[16]	Louwyck A, Vandenbohede A, Bakker M, et al. MODFLOW procedure to simulate axisymmetric flow in radially heterogeneous and layered aquifer systems[J]. Hydrogeology Journal, 2014, 22(5): 1217-1226. doi: 10.1007/s10040-014-1150-0
[17]	董佩, 王旭升. MODFLOW模拟自由面渗流的应用与讨论[J]. 工程勘察, 2009(7): 27-30. https://www.cnki.com.cn/Article/CJFDTOTAL-GCKC200907009.htm Dong P, Wang X S. Application and discussion of MODFLOW's simulation to the seepage of free surface[J]. Journal of Geotechnical Investigation & Surveying, 2009(7): 27-30(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-GCKC200907009.htm
[18]	Hill M C. Preconditioned conjugate-gradient 2 (PCG2), a computer program for solving, ground-water flow equations[R]. Water-Resources Investigations Report 90-4048, Denver: U.S. Geological Survey, 1990.
[19]	Pollock D W. User's Guide for MODPATH/MODPATH-PLOT, Version 3: A Particle tracking post-processing package for MODFLOW, the U.S. Geological survey finite difference groundwater flow model[R]. Open-file report 94-464, Denver: U.S. Geological Survey, 1994.
[20]	陈崇希, 唐仲华, 胡立堂. 地下水流数值模拟理论方法及模型设计[M]. 北京: 地质出版社, 2014. Chen C X, Tang Z H, Hu L T. Theory, method and model design for numerical simulation of groundwater flow[M]. Beijing: Geological Publishing House, 2014 (in Chinese).
[21]	成建梅, 罗一鸣. 岩溶多重介质地下水模拟技术及应用进展[J]. 地质科技通报, 2022, 41(5): 220-229. doi: 10.19509/j.cnki.dzkq.2022.0220 Chen J M, Luo Y M. Overview of groundwater modeling technology and its application in karst areas with multiple-void media[J]. Bulletin of Geological Science and Technology, 2022, 41(5): 220-229(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0220
[22]	郑小康, 杨志兵. 岩溶含水层饱和-非饱和流动与污染物运移数值模拟[J]. 地质科技通报, 2022, 41(5): 357-366. doi: 10.19509/j.cnki.dzkq.2022.0211 Zheng X K, Yang Z B. Numerical simulation of saturated-unsaturated groundwater flow and contaminant transport in a karst aquifer[J]. Bulletin of Geological Science and Technology, 2022, 41(5): 357-366(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0211
[23]	Wen Z, Liu Z T, Jin M G, et al. Numerical modeling of Forchheimer flow to a pumping well in a confined aquifer using the strong-form mesh-free method[J]. Hydrogeology Journal, 2014, 22(5): 1207-1215. doi: 10.1007/s10040-014-1136-y
[24]	Wang Q R, Zhan H B, Tang Z H, et al. Forchheimer flow to a well-considering time-dependent critical radius[J]. Hydrology and Earth System Sciences, 2014, 18(6): 2437-2448. doi: 10.5194/hess-18-2437-2014
[25]	陈崇希, 林敏, 叶善士, 等. 地下水混合井流的理论及应用[M]. 武汉: 中国地质大学出版社, 1998. Chen C X, Lin M, Ye S S, et al. Theory of multi-layer mixed well flow and its application[M]. Wuhan: China University of Geosciences Press, 1998(in Chinese).