Parallel groundwater flow simulation method based on a discrete fracture network model
-
摘要:
裂隙水表现为强烈的非均匀性和各向异性, 而离散裂隙网络模拟方法是目前国际上公认描述裂隙水流动规律最为合理、有效的方法之一。以我国高放废物地质处置地下实验室花岗岩场址为研究对象, 借助高性能数值计算服务器集群及并行程序, 提出了场址岩体离散裂隙网络渗流并行模拟方法。结果表明, 该方法可实现千万级别模型网络单元离散裂隙网络渗流精细化模拟, 提升了程序处理复杂问题的计算效率及能力; 建立了离散裂隙网络模型结构优化与实际情景条件下边界参数赋值方法, 保障了场址水文地质评价过程中不同尺度模型水位信息的有效传递; 模拟区域地下水位沿着裂隙呈网状结构分布, 呈现从南向北流动的趋势, 连通裂隙间的水位呈现从高到低的连续变化, 非连通裂隙的水位是非连续的; 地下水是沿着导水裂隙流动, 裂隙网络的连通性及渗透性对地下水流动特性影响明显。离散裂隙网络渗流并行数值模拟可以更为精细反映裂隙地下水动力场特征, 进一步提升裂隙介质渗流模拟预测能力, 这对深化裂隙水流动规律的认识具有重要意义。
Abstract:Objective Groundwater flow in fractured rocks has strong heterogeneity and anisotropy. The discrete fracture network (DFN) method has been internationally considered as one of the most reasonable and effective methods to describe the fracture water transport.
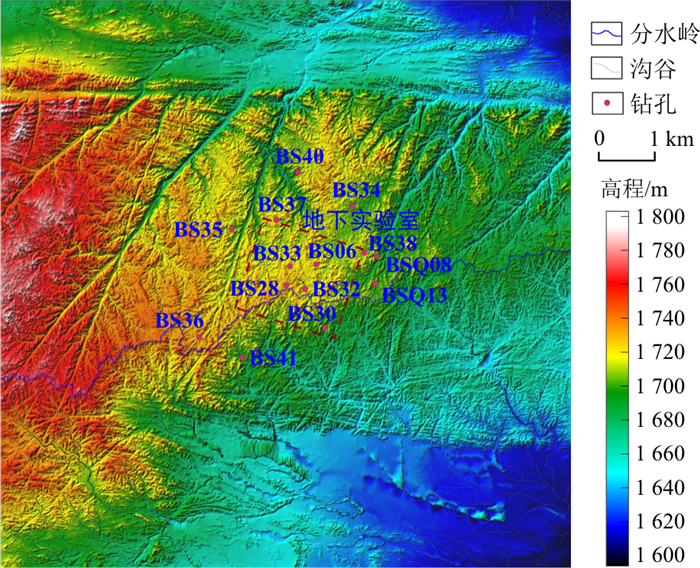
Methods In this work, we focused on granite rock from an underground research laboratory site for the geological disposal of high-level radioactive waste. In addition, a high-performance numerical computing system and parallel codes were employed to develop a groundwater flow simulation method in fractured rocks based on the DFN model.
Results The results indicated that the proposed method could conduct the groundwater flow simulation of DFN with thousands of mesh elements. This improved the computational efficiency and ability of the parallel codes to deal with complex models. We established the DFN model structure optimization and parameter setting methods of boundary conditions in a complex condition. This could ensure that the hydraulic heads were continuous at different scale models. Furthermore, in the model area, the hydraulic head is distributed as a network structure along fractures. For the connected fractures, the water level was continuous and changed from high to low. However, the water level in the nonconnected fractures was discontinuous. Groundwater flows along the fracture from the high water level area to the low water level area. The connectivity and permeability of the fracture network have an obvious influence on the groundwater flow characteristics.
Conclusion Therefore, we could conclude that the parallel groundwater flow simulation method based on the DFN model could more reasonably reflect the groundwater flow in a fractured rock mass. It was of great significance to further improve the simulated prediction ability and deepen the understanding of groundwater flow characteristics in a fractured medium.
-
图 1 研究区地形图及部分钻孔位置
Figure 1. Topographical map of the study area and borehole locations
图 4 裂隙优势产状分组(等角度下半球投影)
Figure 4. Fracture pole orientation of the study area on an equal angle stereonet
图 7 离散裂隙网络模型边界结点赋值结果
Figure 7. Hydraulic heads of boundary nodes for the discrete network fracture model
图 8 离散裂隙网络模型导水系数结果图
Figure 8. Transmissivity setting of the discrete network fracture model
图 9 离散裂隙网络模型水位模拟结果图
Figure 9. Simulated hydraulic head of the discrete network fracture model
图 10 离散裂隙网络模型地下水流速模拟结果
Figure 10. Simulated velocity of the discrete network fracture model
图 11 离散裂隙网络模型溶质运移模拟结果
Figure 11. Mass transport simulated results of the discrete network fracture model
图 12 不同深度离散裂隙网络模型溶质运移模拟结果切面图
Figure 12. Profiles of the mass transport simulated results of the discrete network fracture model at different depths
表 1 裂隙优势产状Elliptical Fisher分布模型参数
Table 1. Elliptical Fisher model parameters for fracture predominarice
优势组编号 优势倾向/(°) 优势倾角/(°) 分布模型 分布模型参数 k R 1 30.21 61.74 Elliptical Fisher 14.50 2.12 2 128.3 60.21 Elliptical Fisher 15.73 1.85 3 230.82 62.55 Elliptical Fisher 17.18 1.83 4 297.59 62.94 Elliptical Fisher 25.16 1.79 5 90.42 81.89 Elliptical Fisher 9.56 48.11 6 165.21 41.65 Elliptical Fisher 15.41 1.83 7 349.46 26.26 Elliptical Fisher 19.68 1.92 8 341.89 63.93 Elliptical Fisher 25.67 2.00 注：表中k为离散系数；R为椭圆模型的长轴与短轴比值 
下载: 导出CSV
表 2 裂隙直径分布模型参数(以自然对数e为底)[42]
Table 2. Fracture size distribution model parameters
优势组编号 分布模型 分布模型参数 平均值μ 方差σ 1 对数正态分布 0.498 5 1.022 0 2 对数正态分布 0.642 7 0.854 4 3 对数正态分布 3.341 0 0.133 2 4 对数正态分布 1.755 0 0.475 6 5 对数正态分布 0.429 9 1.310 0 6 对数正态分布 1.722 0 0.370 4 7 对数正态分布 0.225 1 1.433 0 8 对数正态分布 2.063 0 0.355 9
下载: 导出CSV
表 3 钻孔实测裂隙及模拟结果统计信息
Table 3. Statistical information of borehole fracture logging and simulated results
优势组编号 实测裂隙数量 所占百分比/% 模型中裂隙数量 所占百分比/% 1 67 5.90 365 5.49 2 148 13.03 1 212 18.23 3 137 12.06 227 3.42 4 176 15.49 1 586 23.86 5 94 8.27 376 5.66 6 216 19.01 1 366 20.55 7 141 12.41 209 3.14 8 157 13.82 1 306 19.65
下载: 导出CSV
表 4 不同CPU数量条件下的并行程序执行时间
Table 4. Running times of the parallel code with different CPU numbers
CPU数量 网格剖分时间/s 地下水流数值模拟时间/s 裂隙网格剖分 网格优化 8 980.0 668.1 573.7 16 534.0 675.9 335.5 32 392.0 728.6 217.3 64 315.0 718.2 144.4 96 325.0 764.9 124.0
下载: 导出CSV
-
[1] 郭永海, 王驹, 金远新. 世界高放废物地质处置库选址研究概况及国内进展[J]. 地学前缘, 2001, 8(2): 327-332. https://www.cnki.com.cn/Article/CJFDTOTAL-DXQY200102020.htmGuo Y H, Wang J, Jin Y X. The general situation of geological disposal repository siting in the world and research progress in China[J]. Earth Science Frontiers, 2001, 8(2): 327-332(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DXQY200102020.htm [2] 王驹, 张铁岭, 郑华铃. 世界放射性废物地质处置[M]. 北京: 原子能出版社, 1999.Wang J, Zhang T L, Zheng H L. Geological disposal of radioactive waste in the world[M]. Beijing: Atomic Energy Press, 1999(in Chinese). [3] Bense V F, Gleeson T, Loveless S E, et al. Fault zone hydrogeology[J]. Earth-Science Reviews, 2013, 127: 171-192. doi: 10.1016/j.earscirev.2013.09.008 [4] 张人权, 梁杏, 靳孟贵, 等. 水文地质学基础: 第6版[M]. 北京: 地质出版社, 2011.Zhang R Q, Liang X, Jin M G, et al. Foundation of hydrogeology: 6th Edition[M]. Beijing: Geological Publishing House, 2011(in Chinese). [5] Illman W A. Hydraulic tomography offers improved imaging of heterogeneity in fractured rocks[J]. Groundwater, 2014, 52(5): 659-684. doi: 10.1111/gwat.12119 [6] Neuman S P. Trends, prospects and challenges in quantifying flow and transport through fractured rocks[J]. Hydrogeology Journal, 2005, 13(1): 124-147. doi: 10.1007/s10040-004-0397-2 [7] Gómez-Hernández J J, Cassiraga E F. Theory and practice of sequential simulation[C]//Armstrong M, Dowd P A. Geostatistical simulations. Dordect: Springer, 1994, 7: 111-124. [8] Remy N, Boucher A, Wu J. Applied geostatistics with SGeMS: A user's guide[M]. Cambridge: Cambridge University Press, 2009. [9] Park Y J, Sudicky E A, McLaren R G, et al. Analysis of hydraulic and tracer response tests within moderately fractured rock based on a transition probability geostatistical approach[J]. Water Resources Research, 2004, 40(12): W12404. [10] Blessent D, Therrien R, Lemieux J M. Inverse modeling of hydraulic tests in fractured crystalline rock based on a transition probability geostatistical approach[J]. Water Resources Research, 2011, 47(12): W12530. [11] Zha Y Y, Yeh T C J, Illman W A, et al. What does hydraulic tomography tell us about fractured geological media? A field study and synthetic experiments[J]. Journal of Hydrology, 2015, 531(S1): 17-30. [12] Zha Y, Yeh T C J, Illman W A, et al. Incorporating geologic information into hydraulic tomography: A general framework based on geostatistical approach[J]. Water Resources Research, 2017, 53(4): 2850-2876. doi: 10.1002/2016WR019185 [13] Tiedeman C R, Barrash W. Hydraulic tomography: 3D hydraulic conductivity, fracture network, and connectivity in mudstone[J]. Groundwater, 2019, 58(2): 238-257. [14] Vesselinov V V, Neuman S P, Illman W A. Three-dimensional numerical inversion of pneumatic cross-hole tests in unsaturated fractured tuff: 2. Equivalent parameters, high-resolution stochastic imaging and scale effects[J]. Water Resources Research, 2001, 37(12): 3019-3041. doi: 10.1029/2000WR000135 [15] Berkowitz B. Characterizing flow and transport in fractured geological media: A review[J]. Advances in Water Resources, 2002, 25(8): 861-884. [16] Samardzioska T, Popov V. Numerical comparison of the equivalent continuum, non-homogeneous and dual porosity models for flow and transport in fractured porous media[J]. Advances in Water Resources, 2005, 28(3): 235-255. doi: 10.1016/j.advwatres.2004.11.002 [17] Karimi-Fard M, Gong B, Durlofsky L J. Generation of coarse-scale continuum flow models from detailed fracture characterizations[J]. Water Resources Research, 2006, 42(10): W10423. [18] Cacas M C, Ledoux E, de Marsily G, et al. Modeling fracture flow with a stochastic discrete fracture network: Calibration and validation: 1. The flow model[J]. Water Resources Research, 1990, 26(3): 479-489. [19] Nordqvist A W, Tsang Y, Tsang C, et al. A variable aperture fracture network model for flow and transport in fractured rocks[J]. Water Resources Research, 1992, 28(6): 1703-1713. doi: 10.1029/92WR00216 [20] 宋晓晨, 徐卫亚. 裂隙岩体渗流模拟的三维离散裂隙网络数值模型(Ⅰ): 裂隙网络的随机生成[J]. 岩石力学与工程学报, 2004, 23(12): 2015-2020. https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200412014.htmSong X C, Xu W Y. Numerical model of three-dimensional discrete fracture network for seepage in fractured rocks(Ⅰ): Generation of fracture network[J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23(12): 2015-2020(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200412014.htm [21] 宋晓晨, 徐卫亚. 裂隙岩体渗流模拟的三维离散裂隙网络数值模型(Ⅱ): 稳定渗流计算[J]. 岩石力学与工程学报, 2004, 23(12): 2021-2026. doi: 10.3321/j.issn:1000-6915.2004.12.013Song X C, Xu W Y. Numerical model of three-dimensional discrete fracture network for seepage in fractured rocks(Ⅱ): Computation of steady flow[J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23(12): 2021-2026(in Chinese with English abstract). doi: 10.3321/j.issn:1000-6915.2004.12.013 [22] de Dreuzy J R, Méheust Y, Pichot G. Influence of fracture scale heterogeneity on the flow properties of three-dimensional discrete fracture networks(DFN)[J]. Journal of Geophysical Research: Solid Earth, 2012, 117: B11207. [23] 黄宇, 杨荣森, 韩晓东, 等. 基于定向半变异函数的裂隙网络空间变异性分析与研究[J]. 地质科技通报, 2023, 42(2): 186-193. doi: 10.19509/j.cnki.dzkq.2022.0248Huang Y, Yang R S, Han X D, et al. Analysis and research on spatial variability of fracture network based on oriented semivariogram[J]. Bulletin of Geological Science and Technology, 2023, 42(2): 186-193(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0248 [24] Lang P, Paluszny A, Zimmerman R. Permeability tensor of three-dimensional fractured porous rock and a comparison to trace map predictions[J]. Journal of Geophysical Research: Solid Earth, 2014, 119(8): 6288-6307. doi: 10.1002/2014JB011027 [25] 胡成, 陈刚, 曹孟雄, 等. 基于离散裂隙网络法和水流数值模拟技术的地下水封洞库水封性研究[J]. 地质科技通报, 2022, 41(1): 119-126. doi: 10.19509/j.cnki.dzkq.2022.0029Hu C, Chen G, Cao M X, et al. A case study on water sealing efficiency of groundwater storage caverns using discrete fracture network method and flow numerical simulation[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 119-126(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0029 [26] Koudina N, Garcia R G, Thovert J F, et al. Permeability of three-dimensional fracture networks[J]. Physical Review E, 1998, 57(4): 4466-4479. doi: 10.1103/PhysRevE.57.4466 [27] Bogdanov I, Mourzenko V, Thovert J F, et al. Effective permeability of fractured porous media in steady state flow[J]. Water Resources Research, 2003, 39(1): 1023. [28] Erhel J, De Dreuzy J R, Poirriez B. Flow simulation in three-dimensional discrete fracture networks[J]. SIAM Journal on Scientific Computing, 2009, 31(4): 2688-2705. doi: 10.1137/080729244 [29] Hyman J D, Gable C W, Painter S L, et al. Conforming Delaunay triangulation of stochastically generated three dimensional discrete fracture networks: A feature rejection algorithm for meshing strategy[J]. SIAM Journal on Scientific Computing, 2014, 36(4): A1871-A1894. [30] Ishibashi T, Watanabe N, Hirano N, et al. GeoFlow: A novel model simulator for prediction of the 3-D channeling flow in a rock fracture network[J]. Water resources research, 2012, 48: W07601. [31] Pichot G, Poirriez B, Erhel J, et al. A mortar BDD method for solving flow in stochastic discrete fracture networks[C]// Erhel J, Cander M J, Halpern L, et al. Domain decomposition methods in science and engineering XXI. Cham: Springer, 2014, 98: 99-112. [32] Hyman J D, Karra S, Makedonska N, et al. dfnWorks: A discrete fracture network framework for modeling subsurface flow and transport[J]. Computers & Geosciences, 2015, 84: 10-19. [33] Koch T, Gläser D, Weishaupt K, et al. DuMu(x) 3: An open-source simulator for solving flow and transport problems in porous media with a focus on model coupling[J]. Computers & Mathematics with Applications, 2021, 81(S1): 423-443. [34] Golder Associates Ltd. . FracMan 7.9 Workshop[R]. Buckinghamshire: Golder Associates(UK) Ltd, 2020. [35] Wood A F. ConnectFlow Verification Release 11.4[R]. Oxfordshire: Amec Foster Wheeler, 2016. [36] Hu Y, Xu W, Zhan L, et al. Modeling of solute transport in a fracture-matrix system with a three-dimensional discrete fracture network[J]. Journal of Hydrology, 2021, 605: 127333. [37] Hadgu T, Karra S, Kalinina E, et al. A comparative study of discrete fracture network and equivalent continuum models for simulating flow and transport in the far field of a hypothetical nuclear waste repository in crystalline host rock[J]. Journal of Hydrology, 2017, 553: 59-70. [38] Li X, Li D, Xu Y, et al. A dfn based 3-D numerical approach for modeling coupled groundwater flow and solute transport in fractured rock mass[J]. International Journal of Heat and Mass Transfer, 2020, 149: 119179. [39] Ma G, Li T, Wang Y, et al. Numerical simulations of nuclide migration in highly fractured rock masses by the unified pipe-network method[J]. Computers and Geotechnics, 2019, 111: 261-276. [40] 罗辉, 王驹, 蒋实, 等. 高放废物地质处置新场岩体三维地质模型构建与应用[J]. 物探与化探, 2019, 43(3): 568-575. https://www.cnki.com.cn/Article/CJFDTOTAL-WTYH201903016.htmLuo H, Wang J, Jiang S, et al. Construction and application of three-dimensional geological model in Xinchang block for high-level radioactive waste disposal[J]. Geophysical and Geochemical Exploration, 2019, 43(3): 568-575(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-WTYH201903016.htm [41] Hyman J, Aldrich G, Viswanathan H, et al. Fracture size and transmissivity correlations: Implications for transport simulations in sparse three-dimensional discrete fracture networks following a truncated power law distribution of fracture size[J]. Water Resources Research, 2016, 52(8): 6472-6489. [42] 刘健, 陈亮, 王春萍, 等. 地下实验室围岩力学及长期稳定性研究[R]. 北京: 核工业北京地质研究院, 2019.Liu J, Chen L, Wang C P, et al. Study on mechanical characteristics and long-term stability of surrounding rock of underground research laboratory[R]. Beijing: Beijing Research Institute of Uranium Geology, 2019(in Chinese). [43] Follin S, Hartley L, Rhén I, et al. A methodology to constrain the parameters of a hydrogeological discrete fracture network model for sparsely fractured crystalline rock, exemplified by data from the proposed high-level nuclear waste repository site at Forsmark, Sweden[J]. Hydrogeology Journal, 2014, 22(2): 313-331. [44] Joyce S, Hartley L, Applegate D, et al. Multi-scale groundwater flow modeling during temperate climate conditions for the safety assessment of the proposed high-level nuclear waste repository site at Forsmark, Sweden[J]. Hydrogeology Journal, 2014, 22(6): 1233-1249. [45] 赵敬波, 周志超, 季瑞利, 等. 区域地下水流动模拟与预测评价[R]. 北京: 核工业北京地质研究院, 2020.Zhao J B, Zhou Z C, Ji R L, et al. Numerical model and prediction of regional groundwater flow in Beishan area[R]. Beijing: Beijing Research Institute of Uranium Geology, 2020(in Chinese). [46] Makedonska N, Painter S L, Bui Q M, et al. Particle tracking approach for transport in three-dimensional discrete fracture networks: Particle tracking in 3-D DFNs[J]. Computational Geosciences, 2015, 19(5): 1123-1137. -
下载:
点击查看大图
计量
- 文章访问数: 492
- PDF下载量: 59
- 被引次数: 0
