A comparative study of Kriging and deep learning methods for shallow groundwater level estimation: A case study of the Shenzhen-Shanwei Special Cooperation Zone
-
摘要:
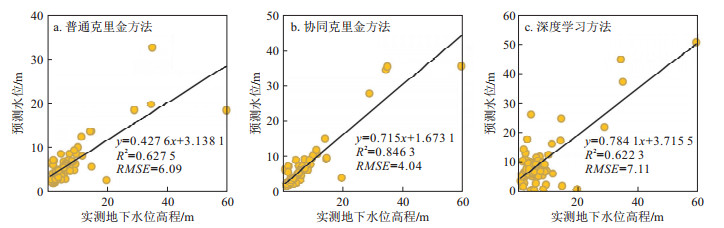
掌握区域地下水位分布是地下水资源评价与环境保护的重要基础。由于区域尺度观测的地下水位数据有限, 克里金插值与深度学习方法逐渐被用于区域地下水位预测, 但两者的适用性及鲁棒性缺乏对比分析。针对这个问题, 基于239口监测井水位, 采用普通克里金方法、融合地表高程的协同克里金方法、深度学习方法估计深汕特别合作区地下水位空间分布, 调查3种方法在区域地下水位预测中的应用潜力。为了研究训练集样本量对3种方法预测效果的影响, 将239口监测井分为2组(76口和163口)用于3种模型的训练。结果显示, 使用76眼井的训练数据拟合验证集样本时
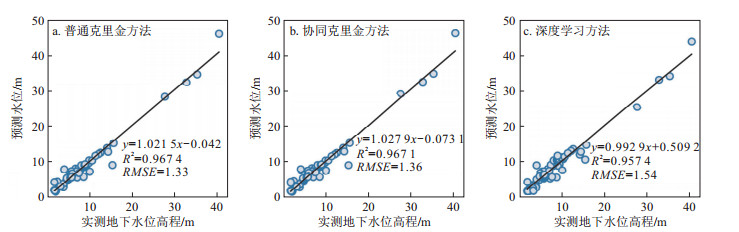
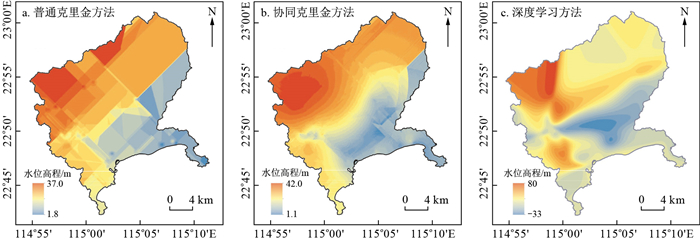
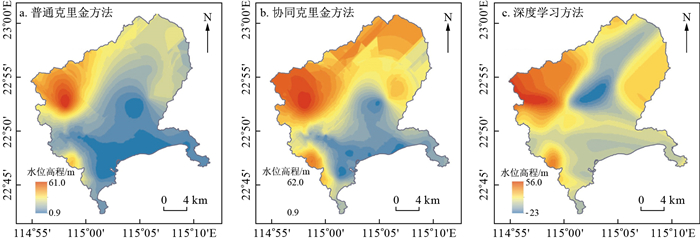
RMSE 分别为6.09, 4.04, 7.11, 考虑了地表高程信息的协同克里金法明显优于普通克里金法与深度学习法。而当训练样本量增加到163口水位数据时, 普通克里金、协同克里金及深度学习法的预测精度都明显提升, 3种方法拟合验证数据集的RMSE 相差很小, 分别为1.33, 1.36, 1.54。另外, 使用较大数据样本量进行全区域的地下水位预测时不同方法得到的预测水位分布均有所提高, 但空间分布特征依旧存在明显差异。结果表明, 当观测数据稀疏时, 融合高程信息的协同克里金方法的预测效果显著高于普通克里金方法和深度学习方法, 而当观测数据量增加到达一定数量时, 3种方法预测得到的RMSE 较接近。Abstract:Objective Knowledge of the regional groundwater level is an important foundation for groundwater resource evaluation and protection. Due to the limited amount of groundwater level data available at the regional scale, Kriging interpolation and deep learning methods are gradually being used for regional groundwater level prediction, but their applicability and robustness lack comparative analysis.
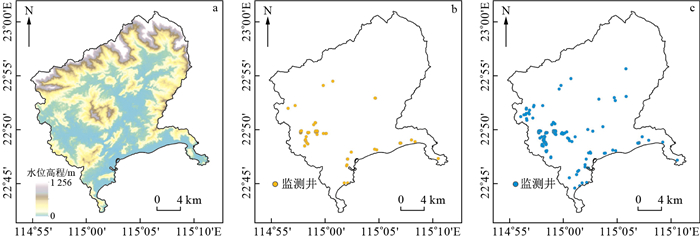
Methods In this paper, spatial interpolation of groundwater levels in the Shenzhen-Shanwei Special Cooperation Zone was carried out using ordinary Kriging, coKriging and deep learning methods to explore the potential of these three methods for practical application to regional groundwater level prediction. To investigate the effect of the training set sample size on the prediction effect of the three methods, 239 monitoring wells were divided into two groups of 76 and 163 wells for the training of the three models.
Results The results showed that the RMSEs were 6.09, 4.04, and 7.11 when the training data of 76 wells were used to fit the validation set, and the Kriging method, which accounts for surface elevation information, was significantly better than the ordinary Kriging method and the deep learning method. In addition, the predicted water level distribution improved when a larger number of samples was used to predict the water level in the region. However, the spatial distribution characteristics still differed significantly.
Conclusion The results show that when the observation data are sparse, the prediction effect of coKriging with elevation information is significantly greater than that of ordinary Kriging and deep learning methods, while the RMSEs obtained by the three methods are similar when the amount of observation data increases to a certain amount.
-
图 1 研究区地表高程图及地下水位监测井分布图
a.深汕合作区地形高程图；b.76眼监测井位置分布；c.163眼监测井位置分布
Figure 1. Ground surface elevations and the distribution of the groundwater level observation wells in the study area
图 3 不同方向地下水位样本变异函数及变异函数模型分析
Figure 3. Sample variogram of groundwater level and variogram model analysis in different directions
图 4 深汕合作区不同方向DEM样本变异函数及变异函数模型分析
Figure 4. Sample variogram of the Shenshan special cooperation zone DEM and variogram model analysis in different directions
图 5 不同方向地下水位同DEM的样本协同变异函数及变异函数模型分析
Figure 5. Sample cross variograms of the groundwater level and DEM(Δh=100 m) and variogram model analysis in different directions
图 6 3种插值方法训练集为76口井的地下水位预测值与实测值对比及相关性分析
Figure 6. Comparison of the predicted and observed groundwater levels by the three methods with a training sample size of 76
图 7 3种插值方法训练集为163口井的地下水位预测值与实测值对比及相关性分析
Figure 7. Comparison of the predicted and observed groundwater levels by the three methods with a training sample size of 163
图 8 3种插值方法训练集为76口井的地下水位空间分布预测结果对比图
Figure 8. Comparison of the distributions of predicted groundwater levels predicted by the three methods with a training sample set of 76
图 9 3种插值方法训练集为163口井的地下水位空间分布预测结果对比图
Figure 9. Comparison of the distributions of predicted groundwater levels predicted by the three methods with a training sample set of 163
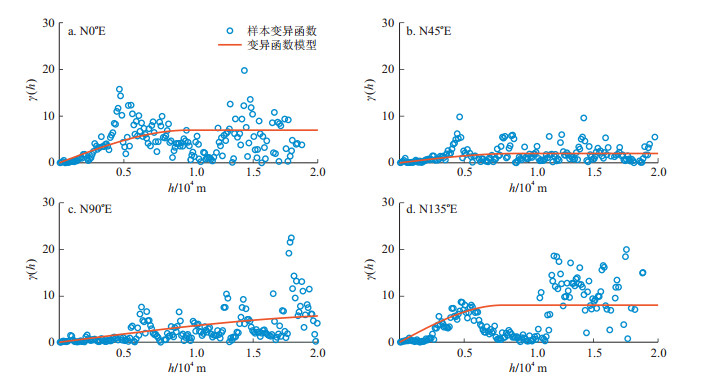
表 1 地下水位样本数据的统计分析结果
Table 1. Statistical results of the observed groundwater level data
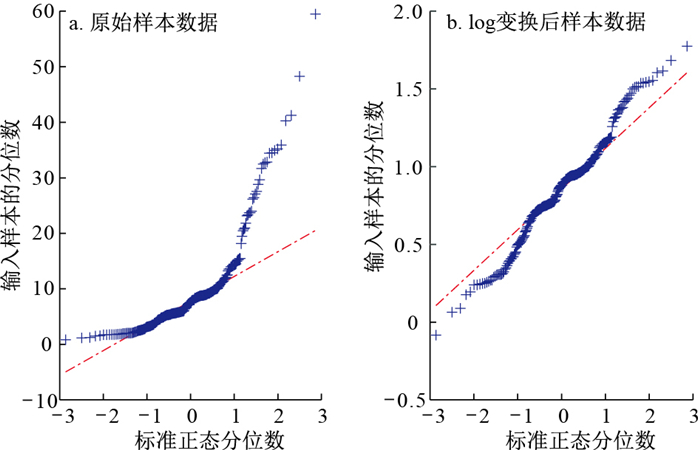
地下水位样本测井/口 最大值/m 最小值/m 均值/m 标准差σ 变差系数Cv 偏度系数 峰度系数 239 59.50 0.83 9.80 8.94 0.91 2.32 6.51 
下载: 导出CSV
表 2 log变换后地下水位样本数据的统计分析结果
Table 2. Statistical results of the observed groundwater level data after log transformation
地下水位样本测井/口 最大值 最小值 均值 标准差σ 变差系数Cv 偏度系数 峰度系数 239 1.77 -0.08 0.85 0.34 0.40 0.05 -0.05
下载: 导出CSV
-
[1] THEODOSSIOU N, LATINOPOULOS P. Evaluation and optimization of groundwater observation networks using the kriging methodology[J]. Environmental Modelling & Software, 2006, 21(7): 991-1000. [2] KUMAR V. Optimal contour mapping of groundwater levels using universal kriging: A case study[J]. Hydrological Sciences Journal, 2007, 52(5): 1038-1050. doi: 10.1623/hysj.52.5.1038 [3] VAROUCHAKIS E A, HRISTOPULOS D T, KARATZAS G P. Improving kriging of groundwater level data using nonlinear normalizing transformations: A field application[J]. Hydrological Sciences Journal, 2012, 57(7): 1404-1419. doi: 10.1080/02626667.2012.717174 [4] VAROUCHAKIS E A, HRISTOPULOS D T. Comparison of stochastic and deterministic methods for mapping groundwater level spatial variability in sparsely monitored basins[J]. Environmental monitoring and Assessment, 2013, 185: 1-19. doi: 10.1007/s10661-012-2527-y [5] BORONINA A, CLAYTON R, GWYNNE C. Recommended methodology for determination of design groundwater levels[J]. Australian Geomechanics Journal, 2015, 50(3): 11-21. [6] SIMO A T G, MARACHE A, LASTENNET R, et al. Geostatistical investigations for suitable mapping of the water table: The Bordeaux case (France)[J]. Hydrogeology Journal, 2016, 24(1): 231. doi: 10.1007/s10040-015-1316-4 [7] ZIRAKBASH T, ADMIRAAL R, BORONINA A, et al. Assessing interpolation methods for accuracy of design groundwater levels for civil projects[J]. Journal of Hydrologic Engineering, 2020, 25(9): 04020042. doi: 10.1061/(ASCE)HE.1943-5584.0001982 [8] DELHOMME J P. Kriging in the hydrosciences[J]. Advances in Water Resources, 1978, 1(5): 251-266. doi: 10.1016/0309-1708(78)90039-8 [9] DUNLAP L E, SPINAZOLA J M. Interpolating water-table altitudes in west-central Kansas using kriging techniques[M]. Department of the Interior, US Geological Survey, 1984. [10] NEUMAN S P, JACOBSON E A. Analysis of nonintrinsic spatial variability by residual kriging with application to regional groundwater levels[J]. Journal of the International Association for Mathematical Geology, 1984, 16: 499-521. doi: 10.1007/BF01886329 [11] AHMADI S H, SEDGHAMIZ A. Geostatistical analysis of spatial and temporal variations of groundwater level[J]. Environmental Monitoring and Assessment, 2007, 129: 277-294. doi: 10.1007/s10661-006-9361-z [12] CHUNG J, ROGERS J D. Interpolations of groundwater table elevation in dissected uplands[J]. Groundwater, 2012, 50(4): 598-607. doi: 10.1111/j.1745-6584.2011.00889.x [13] 王卫光, 薛绪掌, 耿伟. 河套灌区地下水位的空间变异性及其克里金估值[J]. 灌溉排水学报, 2007(1): 18-21. https://www.cnki.com.cn/Article/CJFDTOTAL-GGPS200701004.htmWANG W G, XUE X Z, GENG W. Spatial variability of water level in Hetao Irrigation District of Inner Mongolia and their estimations by the kriging[J]. Journal of Irrigation and Drainage, 2007(1): 18-21. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-GGPS200701004.htm [14] 刘治政, 吴晓东, 林洪孝. Kriging插值模型在地下水位监测网优化中的应用[J]. 人民长江, 2010, 41(9): 14-17. https://www.cnki.com.cn/Article/CJFDTOTAL-RIVE201009005.htmLIU Z Z, WU X D, LIN H X. Kriging interpolation model and its application in optimization of groundwater level monitoring network[J]. Yangtze River, 2010, 41(9): 14-17. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-RIVE201009005.htm [15] 李江, 郝新梅, 范琳琳, 等. 黑河中游绿洲地下水位空间变异性研究[J]. 水力发电学报, 2015, 34(11): 106-115. https://www.cnki.com.cn/Article/CJFDTOTAL-SFXB201511012.htmLI J, HAO X M, FAN L L, et al. Spatial variability of groundwater level in midstream oasis of Heihe River basin[J]. Journal of Hydroelectric Engineering, 2015, 34(11): 106-115. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-SFXB201511012.htm [16] HOEKSEMA R J, CLAPP R B, THOMAS A L, et al. Cokriging model for estimation of water table elevation[J]. Water Resources Research, 1989, 25(3): 429-438. doi: 10.1029/WR025i003p00429 [17] DESBARATS A J, LOGAN C E, HINTON M J, et al. On the kriging of water table elevations using collateral information from a digital elevation model[J]. Journal of Hydrology, 2002, 255(1/4): 25-38. [18] AHMADI S H, SEDGHAMIZ A. Application and evaluation of kriging and cokriging methods on groundwater depth mapping[J]. Environmental Monitoring and Assessment, 2008, 138: 357-368. doi: 10.1007/s10661-007-9803-2 [19] GUZMAN S M, PAZ J O, TAGERT M L M. The use of NARX neural networks to forecast daily groundwater levels[J]. Water Resources Management, 2017, 31: 1591-1603. doi: 10.1007/s11269-017-1598-5 [20] JEONG J, PARK E. Comparative applications of data-driven models representing water table fluctuations[J]. Journal of Hydrology, 2019, 572: 261-273. doi: 10.1016/j.jhydrol.2019.02.051 [21] JEONG J, PARK E, CHEN H, et al. Estimation of groundwater level based on the robust training of recurrent neural networks using corrupted data[J]. Journal of Hydrology, 2020, 582: 124512. doi: 10.1016/j.jhydrol.2019.124512 [22] ZHANG A, WINTERLE J, YANG C. Performance comparison of physical process-based and data-driven models: A case study on the Edwards Aquifer, USA[J]. Hydrogeology Journal, 2020, 28(6): 2025-2037. doi: 10.1007/s10040-020-02169-z [23] MÜLLER J, PARK J, SAHU R, et al. Surrogate optimization of deep neural networks for groundwater predictions[J]. Journal of Global Optimization, 2021, 81: 203-231. doi: 10.1007/s10898-020-00912-0 [24] WUNSCH A, LIESCH T, BRODA S. Groundwater level forecasting with artificial neural networks: A comparison of long short-term memory (LSTM), convolutional neural networks (CNNs), and non-linear autoregressive networks with exogenous input (NARX)[J]. Hydrology and Earth System Sciences, 2021, 25(3): 1671-1687. doi: 10.5194/hess-25-1671-2021 [25] 刘攀, 郑雅莲, 谢康, 等. 水文水资源领域深度学习研究进展综述[J]. 人民长江, 2021, 52(10): 76-83. https://www.cnki.com.cn/Article/CJFDTOTAL-RIVE202110012.htmLIU P, ZHENG Y L, XIE K, et al. Review of research progress in deep learning for hydrology and water resources[J]. Yangtze River, 2021, 52(10): 76-83. (in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-RIVE202110012.htm [26] WUNSCH A, LIESCH T, BRODA S. Deep learning shows declining groundwater levels in Germany until 2100 due to climate change[J]. Nature Communications, 2022, 13(1): 1221. doi: 10.1038/s41467-022-28770-2 [27] ZHANG J, ZHU Y, ZHANG X, et al. Developing a long short-term memory (LSTM) based model for predicting water table depth in agricultural areas[J]. Journal of Hydrology, 2018, 561: 918-929. doi: 10.1016/j.jhydrol.2018.04.065 [28] KUMAR D, ROSHNI T, SINGH A, et al. Predicting groundwater depth fluctuations using deep learning, extreme learning machine and Gaussian process: A comparative study[J]. Earth Science Informatics, 2020, 13: 1237-1250. doi: 10.1007/s12145-020-00508-y [29] TAO H, HAMEED M M, MARHOON H A, et al. Groundwater level prediction using machine learning models: A comprehensive review[J]. Neurocomputing, 2022, 489: 271-308. doi: 10.1016/j.neucom.2022.03.014 [30] 黄宇, 杨荣森, 韩晓东, 等. 基于定向半变异函数的裂隙网络空间变异性分析[J]. 地质科技通报, 2023, 42(2): 186-193. doi: 10.19509/j.cnki.dzkq.2022.0248HUANG Y, YANG R S, HAN X D, et al. Analysis of the spatial variability on a fracture network based on an oriented semivariogram[J]. Bulletin of Geological Science and Technology, 2023, 42(2): 186-193. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.2022.0248 [31] 薛佩佩, 文章, 梁杏. 地质统计学在含水层参数空间变异研究中的应用进展与发展趋势[J]. 地质科技通报, 2022, 41(1): 209-222. 10.19509/j.cnki.dzkq.2022.0015XUE P P, WEN Z, LIANG X. The application progress and development trend of geostatistics in the study of aquifer parameter spatial variation[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 209-222. (in Chinese with English abstract) 10.19509/j.cnki.dzkq.2022.0015 -
下载:
点击查看大图
计量
- 文章访问数: 488
- PDF下载量: 70
- 被引次数: 0
