层级制全局敏感性分析方法及其在地下水模型中的应用

刘玉姣; 戴恒; 李跃东; 崔节波; 文章

doi:10.19509/j.cnki.dzkq.tb20230308

层级制全局敏感性分析方法及其在地下水模型中的应用

doi: 10.19509/j.cnki.dzkq.tb20230308

刘玉姣^{1a, 2,},
戴恒^1b, ,,
李跃东^1b,
崔节波^1a,
文章^1a

1a.
中国地质大学(武汉) 长江流域环境水科学湖北省重点实验室, 武汉 430078
1b.
中国地质大学(武汉) 生物地质与环境地质国家重点实验室, 武汉 430078
2.
生态环境部华南环境科学研究所, 广州 510655

基金项目:

国家自然科学基金项目 42172280

详细信息

作者简介:
刘玉姣, E-mail: Yujiaoliu0122@163.com

通讯作者:
戴恒, E-mail: daiheng@cug.edu.cn

中图分类号: X523
计量
- 文章访问数: 71
- PDF下载量: 3
- 被引次数: 0
出版历程
- 收稿日期: 2023-05-31
- 录用日期: 2023-06-29
- 修回日期: 2023-06-28

Method of hierarchical global sensitivity analysis and its application in groundwater models

LIU Yujiao^{1a, 2
,},
DAI Heng^{1b
, ,},
LI Yuedong^1b,
CUI Jiebo^1a,
WEN Zhang^1a

1a.
Hubei Key Laboratory of Yangtze Catchment Environmental Aquatic Science, China University of Geosciences(Wuhan), Wuhan 430078, China
1b.
State Key Laboratory of Biogeology and Environmental Geology, China University of Geosciences(Wuhan), Wuhan 430078, China
2.
South China Institute of Environmental Science, Ministry of Ecological Environment, Guangzhou 510655, China

More Information

Author Bio:
LIU Yujiao, E-mail: Yujiaoliu0122@163.com

Corresponding author: DAI Heng, E-mail: daiheng@cug.edu.cn

摘要

摘要:
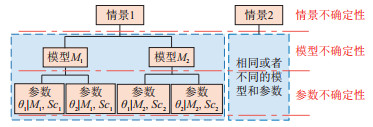
在地下水建模中, 为了更好地利用有限的资金和资源去最优化地降低预测结果的不确定性, 需要使用敏感性分析来测算各个模型输入因素的重要性。提出了改进的层级制全局敏感性分析方法来量化不同类型的输入不确定性对输出结果不确定性的贡献, 并以此评估地下水模型中各个不确定性过程对输出结果的影响程度; 使用一个理想的地下水污染运移模拟模型作为算例对该新方法进行了测试和展示。结果表明, 模型的不确定性是该案例预测结果不确定性的主要来源, 而且地质模型的不确定性相较于其他模型更为重要。该方法能够为地下水模型提供更加全面的敏感性分析, 相较于传统的参数敏感性分析, 新方法能够考虑的不确定性输入因素更多, 计算效率明显提升, 可为模型使用者和管理者提供更有用的敏感性信息。
- 地下水模型 /
- 不确定性 /
- 全局敏感性分析 /
- 层级制全局敏感性分析
Abstract: Objective
Sensitivity analysis is an crucial tool in groundwater modelling for measuring the importance of various model inputs, enabling better allocation of limited funds and resources to reduce predictive uncertainty.
Methods
In this paper, we propose an enhanced hierarchical global sensitivity analysis method to quantify contribution of different types of input uncertainty to model outputs, and to assess the impact of each uncertain process on groundwater model predictions. To test and demonstrate the new method, a hypothetical case study of groundwater flow and contaminant transport is used to validate.
Results
The results indicate that model uncertainty is the main source of prediction uncertainty in this case, and uncertainty from the geological model is more important than that of other models.
Conclusion
The proposed method offers a more comprehensive sensitivity analysis for groundwater models. Compared with traditional parameter sensitivity analysis, the new method can consider more uncertain input factors, significantly improve computational efficiency, and provide more useful sensitivity information for model users and managers.
- groundwater model /
- uncertainty /
- global sensitivity analysis /
- hierarchical global sensitivity analysis
注释:

所有作者声明不存在利益冲突。

HTML全文

图 1 多层结构的层级制不确定性分析框架

M为模型集中包含的所有模型；θ为参数集中包含的所有参数；Sc为集合中包含的所有不确定的情景

Figure 1. Hierarchical uncertainty analysis framework for multi-layer structures

下载: 全尺寸图片幻灯片

图 2 潜水含水层一维地下水流示意图

x为横向位置, m；L为河间地块横向的长度, m；其他参数见正文

Figure 2. Schematic diagram of one-dimensional groundwater flow model in an unconfined aquifer

下载: 全尺寸图片幻灯片

图 3 5 400 m处3种情景下8个模型的乙烯质量浓度预测结果的概率密度曲线(PDFs) (实线为均质模型，虚线为非均质模型)

Figure 3. Probability density functions(PDFs) of the ethene concentrations at 5 400 m for the 8 models under 3 scenarios, respectively

下载: 全尺寸图片幻灯片

图 4 5 400 m处3种情景下对8个模型预测结果平均后预测乙烯质量浓度的概率密度曲线(PDFs)

Figure 4. Probability density functions(PDFs) of ethene concentrations at 5 400 m using the average of 8 models under 3 scenarios, respectively

下载: 全尺寸图片幻灯片

图 5 不同的模型运算执行次数下5 400 m处乙烯质量浓度的3种敏感度系数的计算结果

Figure 5. Three sensitivity indices of ethene concentration at 5 400 m under different number of model executions

下载: 全尺寸图片幻灯片

图 6 预测结果总方差的逐层分解图(5 400 m处)

Figure 6. Variance decomposition of the total variance of prediction results at 5 400 m

下载: 全尺寸图片幻灯片

图 7 3种情景下的模型内方差分解结果

Figure 7. Decomposition of within model variance under 3 scenarios

下载: 全尺寸图片幻灯片

图 8 3种情景下8个模型在5 100~5 700 m内所有空间位置计算的乙烯质量浓度均值

Figure 8. Mean of ethene concentration calculated by 8 models at all locations from 5 100 to 5 700 m under 3 scenarios respectively

下载: 全尺寸图片幻灯片

图 9 3种敏感度系数随空间位置的变化图

Figure 9. Variation of three sensitivity indices with spatial locations

下载: 全尺寸图片幻灯片

图 10 对5 100~5 700 m范围内各位置输出结果的总方差进行逐层分解的结果

Figure 10. Variance decomposition of the total variance at each locations from 5 100 to 5 700 m

下载: 全尺寸图片幻灯片

图 11 6种过程子模型的敏感度系数随空间位置的变化

地质模型(G₁, G₂)、入渗补给模型(R₁, R₂)和融雪模型(SN₁, SN₂)

Figure 11. Variation of sensitivity indices of the 6 models of all processes

下载: 全尺寸图片幻灯片

参考文献(26)

[1]	SONG X M, ZHANG J Y, ZHAN C S, et al. Global sensitivity analysis in hydrological modeling: Review of concepts, methods, theoretical framework, and applications[J]. Journal of Hydrology, 2015, 523: 739-757. doi: 10.1016/j.jhydrol.2015.02.013
[2]	郑小康, 杨志兵. 岩溶含水层饱和-非饱和流动与污染物运移数值模拟[J]. 地质科技通报, 2022, 41(5): 357-366. doi: 10.19509/j.cnki.dzkq.2022.0211 ZHENG X K, YANG Z B. Numerical simulation of saturated-unsaturated groundwater flow and contaminant transport in a karst aquifer[J]. Bulletin of Geological Science and Technology, 2022, 41(5): 357-366. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.2022.0211
[3]	董贵明, 王颖, 詹红兵, 等. 二维承压非稳定流水均衡区间的数值模拟[J]. 地质科技通报, 2023, 42(4): 75-82. doi: 10.19509/j.cnki.dzkq.tb20230028 DONG G M, WANG Y, ZHAN H B, et al. Numerical simulation of the water budget interval for unsteady two-dimensional confined flow[J]. Bulletin of Geological Science and Technology, 2023, 42(4): 75-82. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.tb20230028
[4]	SALTELLI A, ALEKSANKINA K, BECKER W, et al. Why so many published sensitivity analyses are false: A systematic review of sensitivity analysis practices[J]. Environmental Modelling & Software, 2019, 114: 29-39.
[5]	宋美钰, 施小清, 康学远, 等. DNAPL场地污染通量升尺度预测的敏感性分析[J]. 地质科技通报, 2023, 42(2): 327-335. doi: 10.19509/j.cnki.dzkq.tb20220262 SONG M Y, SHI X Q, KANG X Y, et al. Sensitivity analysis of upscaling prediction of the mass flux at DNAPL contaminated sites[J]. Bulletin of Geological Science and Technology, 2023, 42(2): 327-335. (in Chinese with English abstract) doi: 10.19509/j.cnki.dzkq.tb20220262
[6]	MAI J, CRAIG J R, TOLSON B A. Simultaneously determining global sensitivities of model parameters and model structure[J]. Hydrology and Earth System Sciences, 2020, 24(12): 5835-5858. doi: 10.5194/hess-24-5835-2020
[7]	SALTELLI A, ANNONI P, AZZINI I, et al. Variance based sensitivity analysis of model output: Design and estimator for the total sensitivity index[J]. Computer Physics Communications, 2010, 181(2): 259-270. doi: 10.1016/j.cpc.2009.09.018
[8]	PERZAN Z, BABEY T, CAERS J, et al. Local and global sensitivity analysis of a reactive transport model simulating floodplain redox cycling[J]. Water Resources Research, 2021, 57(12): 1-24.
[9]	DELL'OCA A, RIVA M, GUADAGNINI A. Moment-based metrics for global sensitivity analysis of hydrological systems[J]. Hydrology and Earth System Sciences, 2017, 21(12): 6219-6234. doi: 10.5194/hess-21-6219-2017
[10]	HÖGE M, GUTHKE A, NOWAK W. The hydrologist's guide to Bayesian model selection, averaging and combination[J]. Journal of Hydrology, 2019, 572: 96-107. doi: 10.1016/j.jhydrol.2019.01.072
[11]	刘玉珍, 王本德, 姜英震. 基于可变水文地质参数的地下水系统数学模型[J]. 水科学进展, 2009, 20(3): 398-402. LIU Y Z, WANG B D, JIANG Y Z. Mathematical model for groundwater system based on the variable hydro-geological parameters[J]. Advances in Water Science, 2009, 20(3): 398-402. (in Chinese with English abstract)
[12]	施小清, 吴吉春, 吴剑锋, 等. 多个相关随机参数的空间变异性对溶质运移的影响[J]. 水科学进展, 2012, 23(4): 509-515. SHI X Q, WU J C, WU J F, et al. Effects of the heterogeneity of multiple correlated random parameters on solute transport[J]. Advances in Water Science, 2012, 23(4): 509-515. (in Chinese with English abstract)
[13]	史良胜, 杨金忠, 林琳, 等. 基于尺度因素的地下水随机分析[J]. 水科学进展, 2007, 18(1): 39-43. SHI L S, YANG J Z, LIN L, et al. Stochastic analysis of groundwater based on scale factor[J]. Advances in Water Science, 2007, 18(1): 39-43. (in Chinese with English abstract)
[14]	DAI H, YE M. Variance-based global sensitivity analysis for multiple scenarios and models with implementation using sparse grid collocation[J]. Journal of Hydrology, 2015, 528: 286-300.
[15]	曾献奎, 王栋, 吴吉春. 地下水流概念模型的不确定性分析[J]. 南京大学学报(自然科学版), 2012, 48(6): 746-752. ZENG X K, WANG D, WU J C. Uncertainty analysis of groundwater flow conceptual model[J]. Journal of Nanjing University(Natural Sciences), 2012, 48(6): 746-752. (in Chinese with English abstract)
[16]	DELL'OCA A, RIVA M, GUADAGNINI A. Global sensitivity analysis for multiple interpretive models with uncertain parameters[J]. Water Resources Research, 2020, 56(2): 1-20. http://www.xueshufan.com/publication/3004180945
[17]	MAI J, TOLSON B A. Model variable augmentation(MVA) for diagnostic assessment of sensitivity analysis results[J]. Water Resources Research, 2019, 55(4): 2631-2651.
[18]	DAI H, CHEN X Y, YE M, et al. Using Bayesian networks for sensitivity analysis of complex biogeochemical models[J]. Water Resources Research, 2019, 55(4): 3541-3555.
[19]	BIANCHI JANETTI E, GUADAGNINI L, RIVA M, et al. Global sensitivity analyses of multiple conceptual models with uncertain parameters driving groundwater flow in a regional-scale sedimentary aquifer[J]. Journal of Hydrology, 2019, 574: 544-556.
[20]	MAI J, CRAIG J R, TOLSON B A, et al. The sensitivity of simulated streamflow to individual hydrologic processes across North America[J]. Nature Communications, 2022, 13(1): 455.
[21]	LIU H F, DAI H, NIU J, et al. Hierarchical sensitivity analysis for a large-scale process-based hydrological model applied to an Amazonian watershed[J]. Hydrology and Earth System Sciences, 2020, 24(10): 4971-4996.
[22]	DRAPER D, PEREIRA A, PRADO P, et al. Scenario and parametric uncertainty in GESAMAC: A methodological study in nuclear waste disposal risk assessment[J]. Computer Physics Communications, 1999, 117(1/2): 142-155.
[23]	DRAPER D. Assessment and propagation of model uncertainty[J]. Journal of the Royal Statistical Society Series B(Statistical Methodology), 1995, 57(1): 45-70.
[24]	HOCK R. Temperature index melt modelling in mountain areas[J]. Journal of Hydrology, 2003, 282(1/4): 104-115.
[25]	KUSTAS W P, RANGO A, UIJLENHOET R. A simple energy budget algorithm for the snowmelt runoff model[J]. Water Resources Research, 1994, 30(5): 1515-1527.
[26]	DAI H, ZHANG F Q, YE M, et al. A computationally efficient method for estimating multi-model process sensitivity index[J]. Water Resources Research, 2022, 58(10): 1-21.